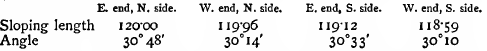Section 5.8.1
It is not obviously clear why the chamber is called "The Queen´s Chamber", as there are no traces or hints referring to any queen. Anyway, there is a global consensus to call the chamber "The Queen´s Chamber", so I do it as well.
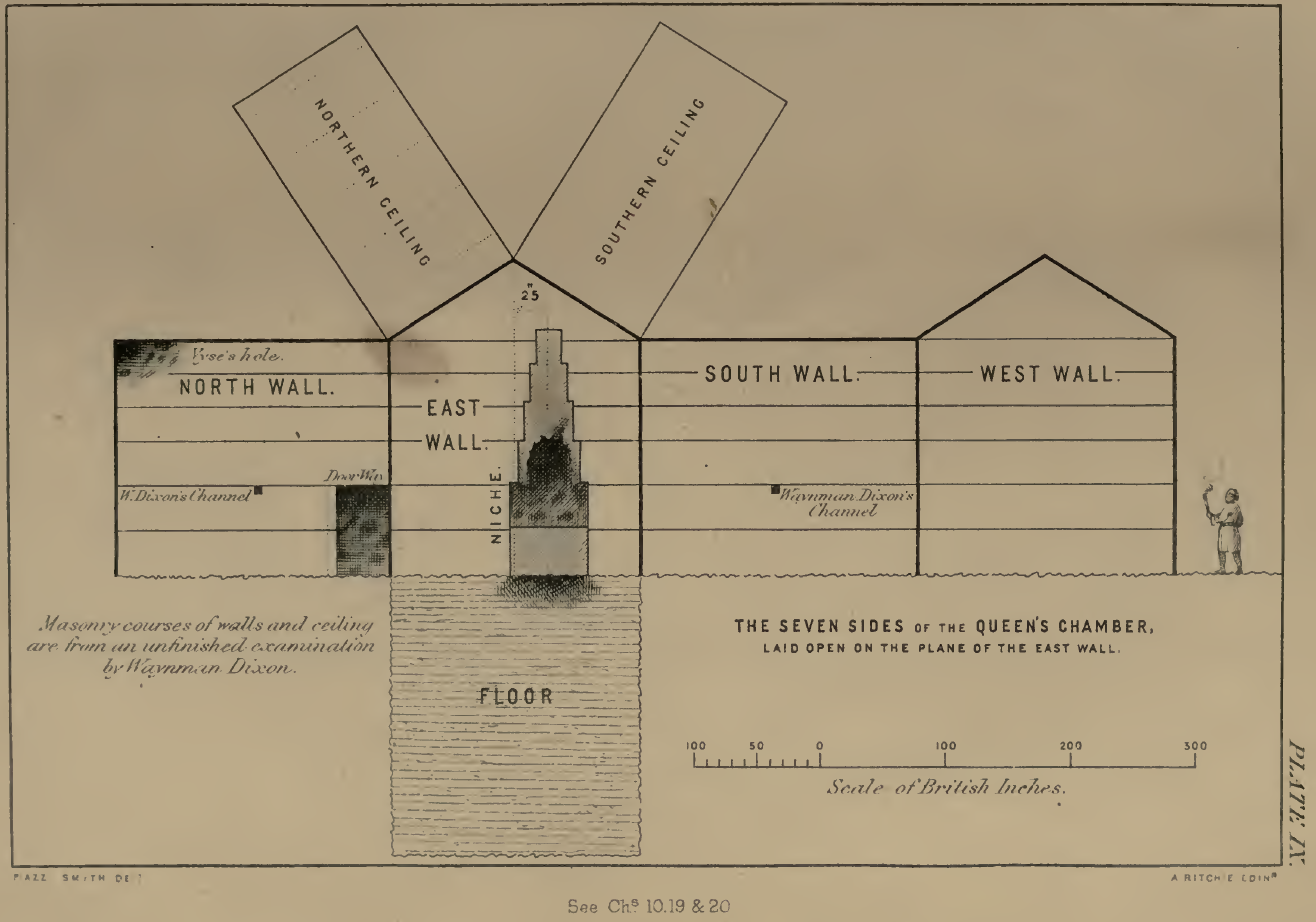
The chamber is empty, it has an angular roof and the eastern wall has niche with four overlappings.
The walls, roof and niche is made of fine white limestone, which is not a part of the masonry in general. The floor is a part of the masonry, very rough and uneven. The chamber has no "real floor" and has the same level as the lower part of the floor in the horizontal passageway.
The greater part of the walls is covered with salt incrustation, which made it hard to find clear joints between the stones. The joints are filled with cement, which is common in the masonry.
Here is a picture of the niche on the eastern wall and the doorway in the northern wall:Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910 , (page 296)
It looks like the northern and the eastern parts of the niche have been "examined" in a destructive way.
Section 5.8.2
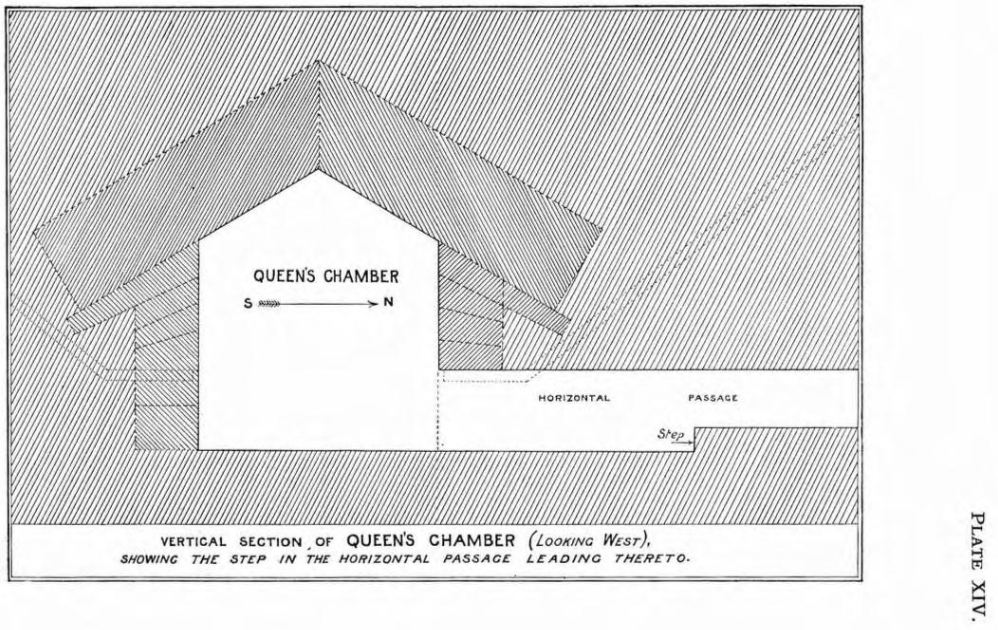
Edgar made a drawing of the chamber seen from East towards West:Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910 , (page 53)
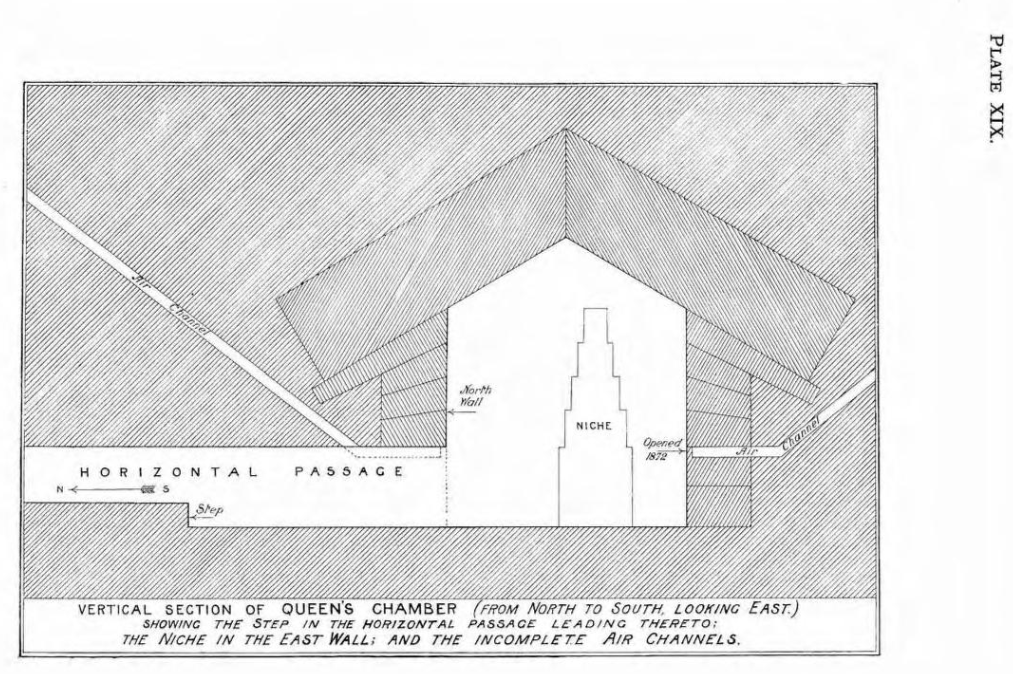
Here is another drawing made by Edgar showing the chamber towards East:
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910 , (page 70)
The two channels are also shown leading or pointing to the outside of the pyramid.
The southern and northern walls are shown with angled stones in a great part of the walls.Section 5.8.3
The northern channel was opened in 1872 and has a rectangular shape. Here is a picture of the hole taken by Edgar:Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910 , (page 298)
Section 5.8.4
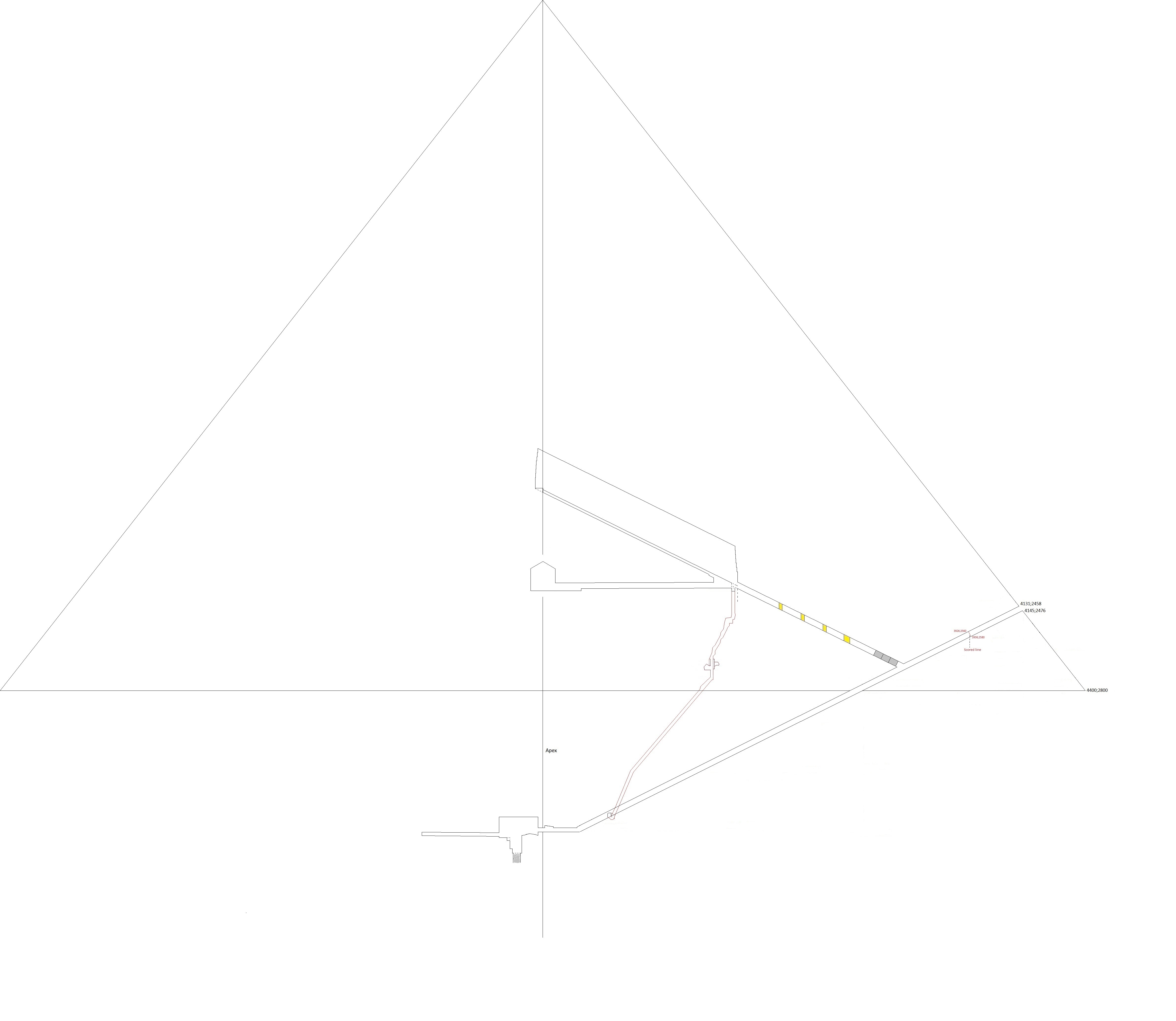
The overview is extended with the Queen´s chamber:As you can see, the Queen´s chamber is the only (known) chamber in the Great Pyramid which is located exactly in the midline of the south-north direction.
Petrie did the same observation and added some measures:
"41. In the Queen's Chamber it seems, from the foregoing statement, that the ridge of the roof is exactly in the mid-place of the Pyramid, equidistant from N. and S. sides ; it only varies from this plane by a less amount than the probable error of the determination.
The size of the chamber (after allowing suitably in each part for the incrustation of salt) is on an average 205,85 wide, and 226,47 long, 184,47 high on N. and S. walls, and 245,1 high to the top of the roof ridge on E. and W. walls. The variations of the horizontal quantities in detail are as follows, from the mean dimensions."
(S5orig-[S41]-P66-L29-37)
My comments:
The above mentioned measures are in British inches.
205,85" = 9,98 cubits RM (average) wide, which equals 100 pixels. (The nominal measure might have been 10 cubits NM).
226,47" = 10,98
cubits RM (average) long * , which equals 110 pixels.
(The nominal measure might have been 11 cubits NM).
184,47" = 8,95
cubits RM (average) high on northern and southern walls ** , which equals 90 pixels.
(The nominal measure might have been 9 cubits NM).
245,1" = 11,89
cubits RM (average) high to the top of the roof ridge, which equals 119 pixels.
(The nominal measure is unknown).
Petrie informed optional lengths of the chamber:
"Beside the above resulting length of the middle of the chamber on the floor, separate measures were taken on the two walls; these give N. 227.41, middle (from above) 227.47, S. 227.61 ; mean of all 227.50 for floor length."
(S5orig-[S42]-P67-L28-31)
My comments to the measured length of the chamber:
227,41" = 11,03 cubits RM equal to 110 pixels, (on northern wall at the floor).
227,47" = 11,03 cubits RM equal to 110 pixels, (middle at the ridge).
227,61" =
11,04 cubits RM equal to 110 pixels, (on southern wall at the floor).
227,50" = 11,03 cubits RM equal to 110 pixels, mean value.
Petrie measured the angled roof stones as well:
(S5orig-[S42]-P69-L10-12)
My comments:
The mentioned sloping calculated lengths are in
British inches.
Northern side:
120,00" = 5,82 cubits RM, east end on north side.
119,96" = 5,82 cubits RM, west end on north side.
Average = 5,82 cubits on northern side.
Southern side:
119,12" = 5,78 cubits RM, east end on south side.
118,59" = 5,75 cubits RM, west end on south side.
Average = 5,76 cubits on southern side.
The angles:
Northern side:
30° 48´ = 30,80° , east end on north side.
30° 14´ = 30,23° , west end on north side.
Average = 30,52° on northern side.
Southern side:
30° 33´ = 30,55° , east end on south side.
30° 10´ = 30,17° , west end on south side.
Average = 30,36° on southern side.
Section 5.8.5
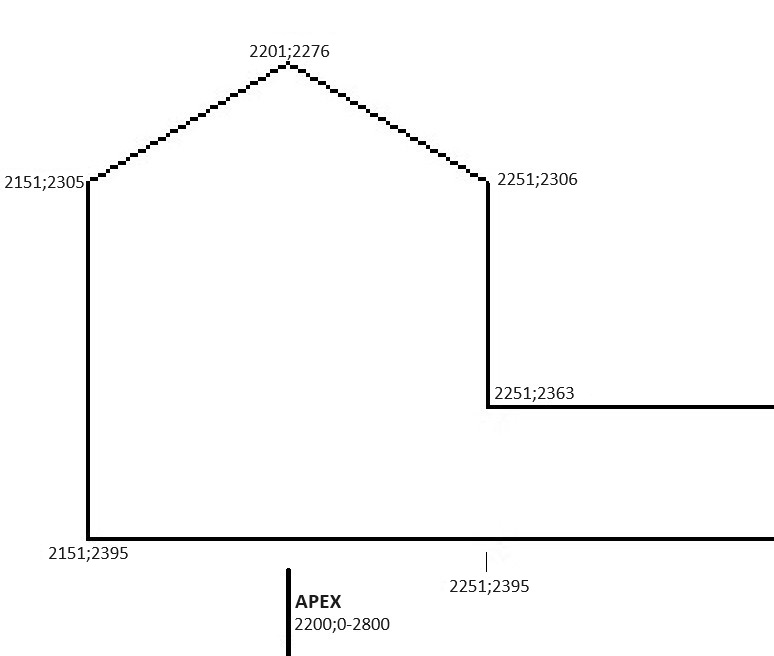
The coordinates are:Calculations based on the lengths in section 5.8.4:
(2251 - 100);2395 = 2151;2395, (southern corner at floor).
2151;(2395 - 90) = 2151;2305, (southern corner at roof).
(2151 +50);(2395 - 119) = 2201; 2276, (roof ridge, mid of chamber).
2251;(2363 -57) = 2251;2306, (northern corner at roof).
The floor consists of raw masonry stones in the Queen´s chamber and the lower parts of the horizontal passageway. Measuring the heights was difficult as the floor surface is uneven and rough. Petrie saw this and mentioned:
"42. In the matter of height, the courses vary a good deal ; and far more care was spent on the closeness, than on the regularity of the joints. For a starting point in measurement, the general floor is hopelessly irregular, consisting plainly of" rough core masonry ; and furthermore, it has been built over with similar rough masonry, which was afterwards stripped down to insert the chamber walls. This is proved by there being no fewer than eight edges of sunken spaces upon it, made (according to the universal habit of pyramid builders) to let in the inequalities of the upper course into the surface of the course below it. These sunken edges are well seen in other parts of the core masonry, and their meaning here is unequivocal. But all round the chamber, and the lower part of the passage leading to it, is a footing of fine stone, at the rough floor level ; this projects I to 4 inches from the base of the walls, apparently as if intended as a support for flooring blocks, which have never been introduced. It is to this footing, or ledge that we must refer as the starting point.."(S5orig-[S42]-P67-L18-16 and P68-L1-5)
The footing can be seen clearly in the horizontal passageway:
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910 , revised by me.
Section 5.8.6
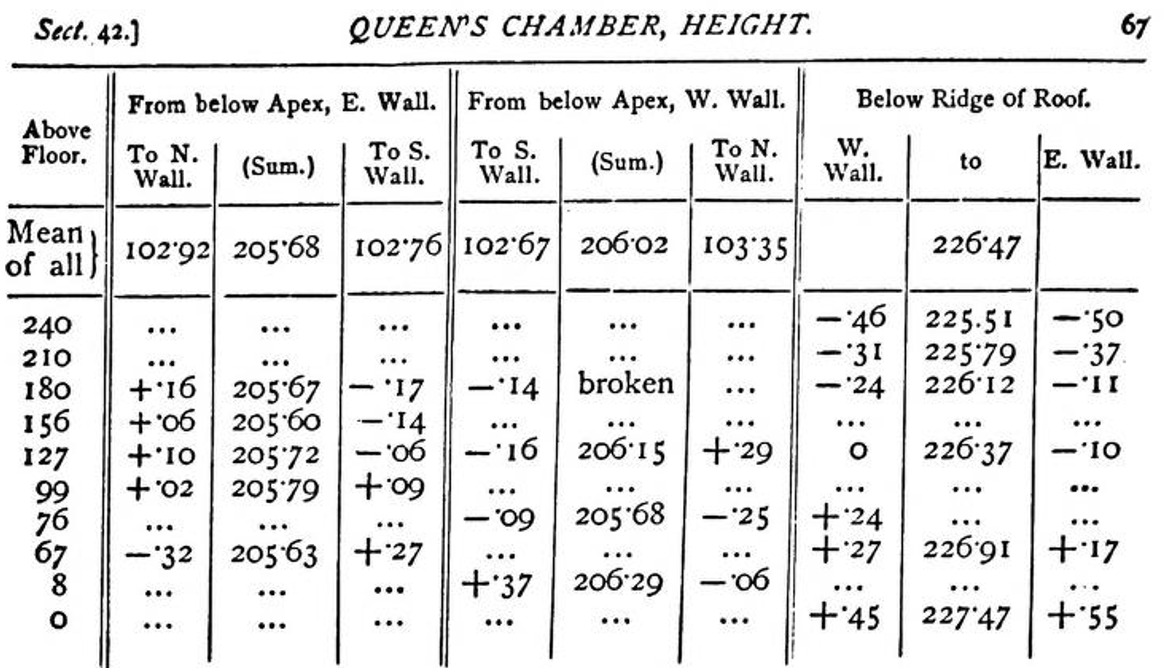
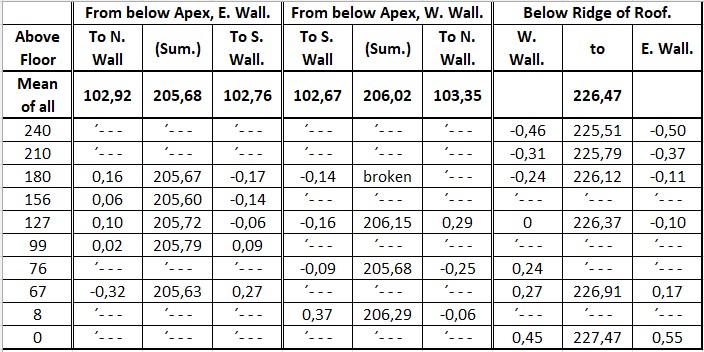
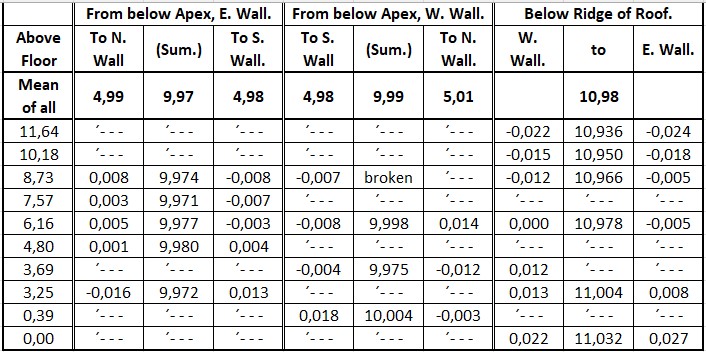
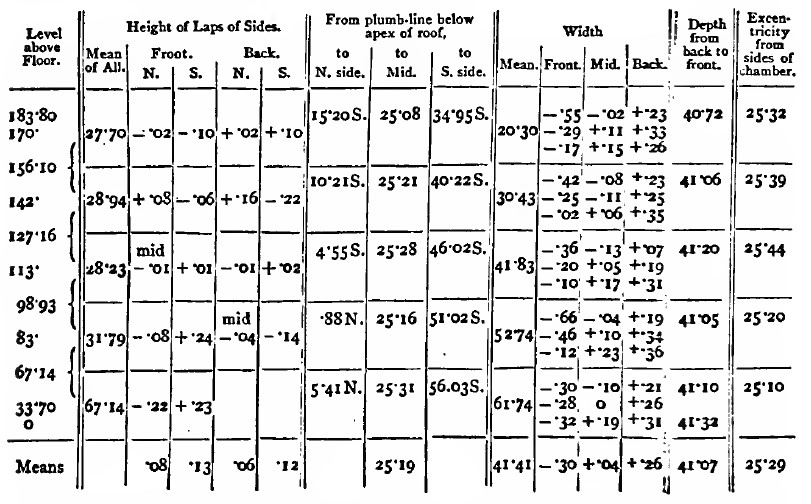
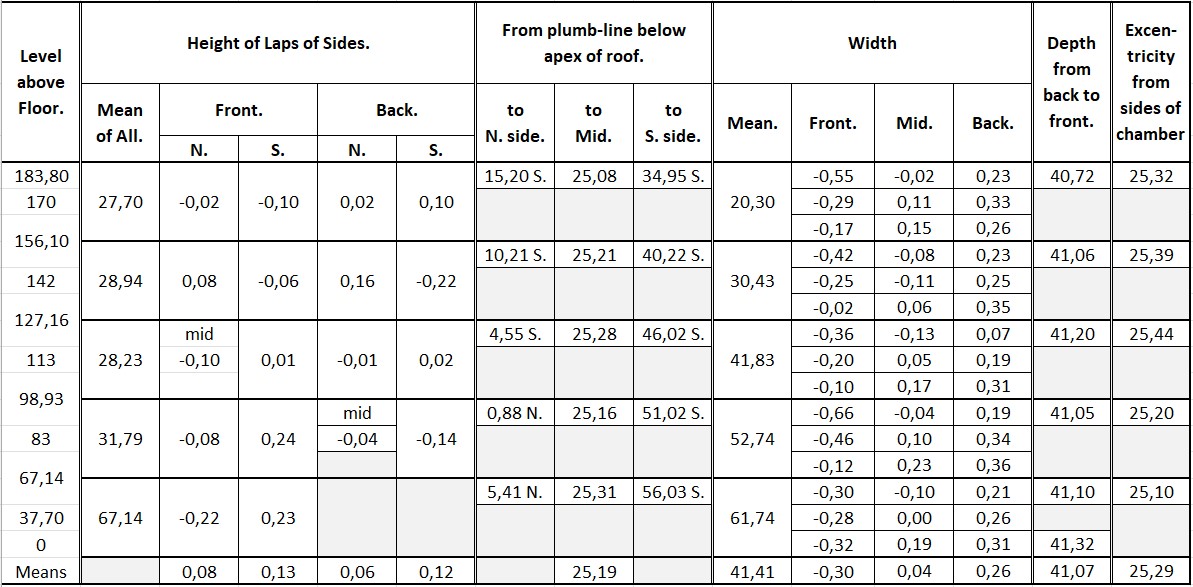
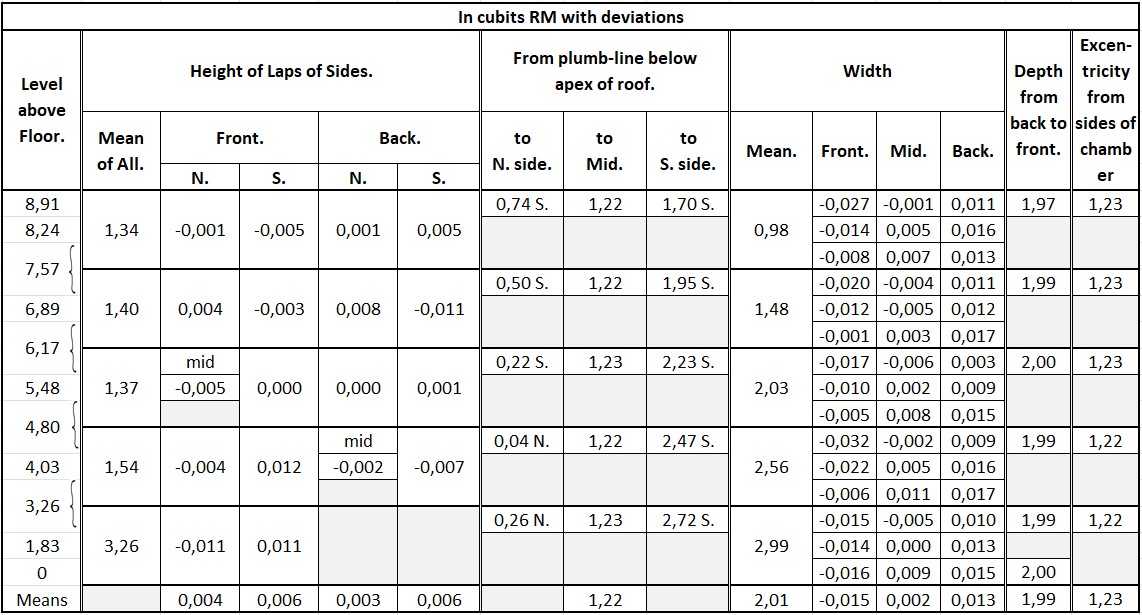
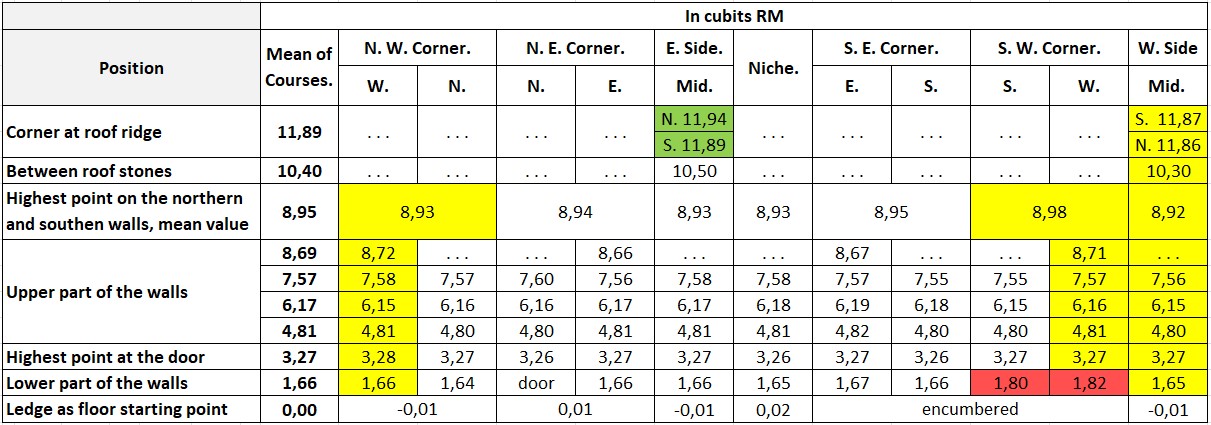
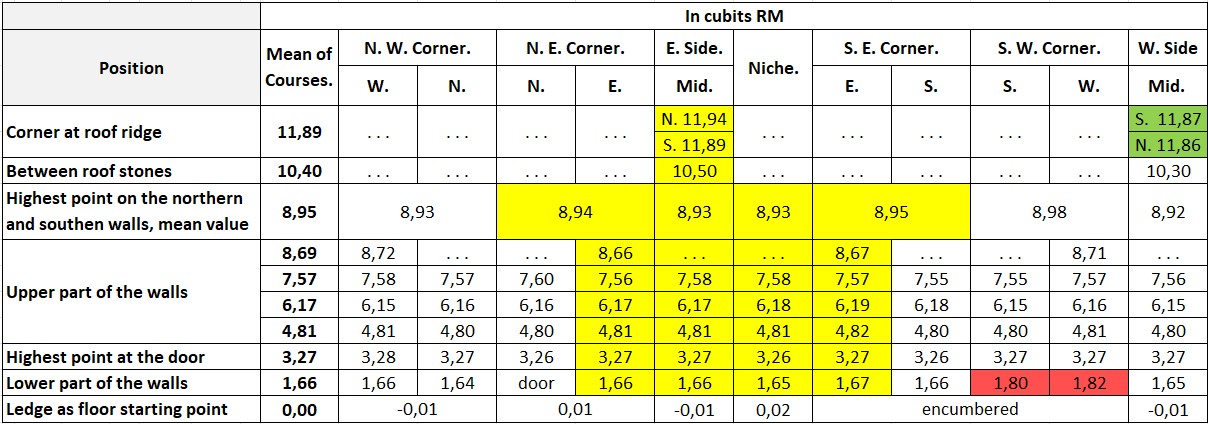
When Petrie observed small differences of the measured lengths in the east-west direction, he decided to measure the width of the chamber from the middle of the chamber (following the roof ridge) to the northern wall respectively the southern wall:(S5orig-[S42]-P67-table)
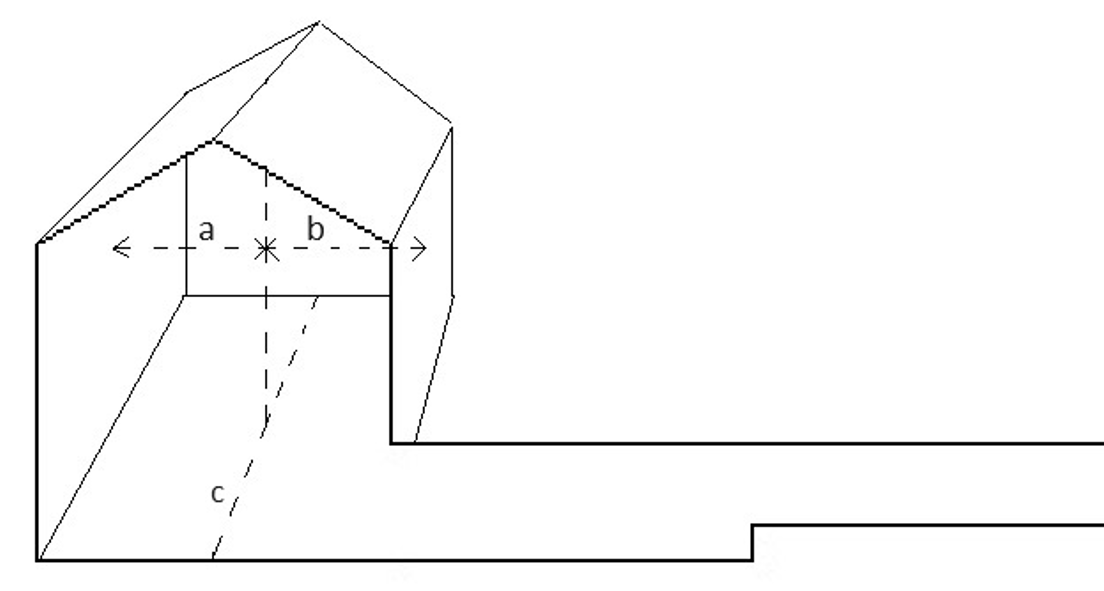
This table might be a bit tricky to understand, so below I have made a drawing to make it more clear:
The columns consists of:
"Above floor" : The measured width at a certain height.
"From below Apex , E. Wall" , "To N. Wall" : It is the length "b" at the eastern wall , (the wall closest to the viewer).
"From below Apex , E. Wall" , "To S. Wall" : It is the length "a" at the eastern wall, (the wall closest to the viewer).
"From below Apex , W. Wall" , "To S. Wall" :
It is the length "a" at the western wall, (the wall far away from the viewer).
"From below Apex , W. Wall" , "To N. Wall" :
It is the length "b" at the western wall, (the wall far away from the viewer).
"Below Ridge of Roof", "W. Wall" : It is the length "c" at the western wall , (the wall far away from the viewer).
"Below Ridge of Roof", "E. Wall" :
It is the length "c" at the eastern wall
,
(the wall closest to the viewer).
The figures in the two columns " From below Apex , E. Wall" and "From below Apex , E. Wall" are results of the same kind of measurement, from the middle of the chamber, but in the opposite direction of each other. The sub column " Sum" is just the lengths "a" and "b" added to each other. Petrie did not inform about the real measures in the two sub columns as he was focused on the deviations. For example; at "180" "Above Floor" in the section "From below Apex , E. Wall" , sub column"To N. Wall" he could have informed 103,08 ( =102,92 + 0.16), (the mean value 102,92 plus the deviation 0,16 in inches), and in the sub column "To S. Wall" he could have written 102,59 ( =102,76 - 0.17) (inches). The sum of 103,08 and 102,59 is 205,67, which you can read in the table.
The figures in the column "Below ridge of Roof" are results of complete real measurements from the western wall to the eastern wall. Again the deviations are calculated. For example;
at "240" "Above Floor" in the section
"Below ridge of Roof" in the sub column "W. Wall" , Petrie could have mentioned the real measure 112,78 ( =226.47/2-0,46) and
in the sub column "E. Wall" , Petrie could have mentioned the real measure 112,74 ( 226,47/2-0,50). The sum of 112,78 and 112,74 is 225,52 and not 225,51 as in Petries table. Might be a rounding error - somewhere.
Section 5.8.7
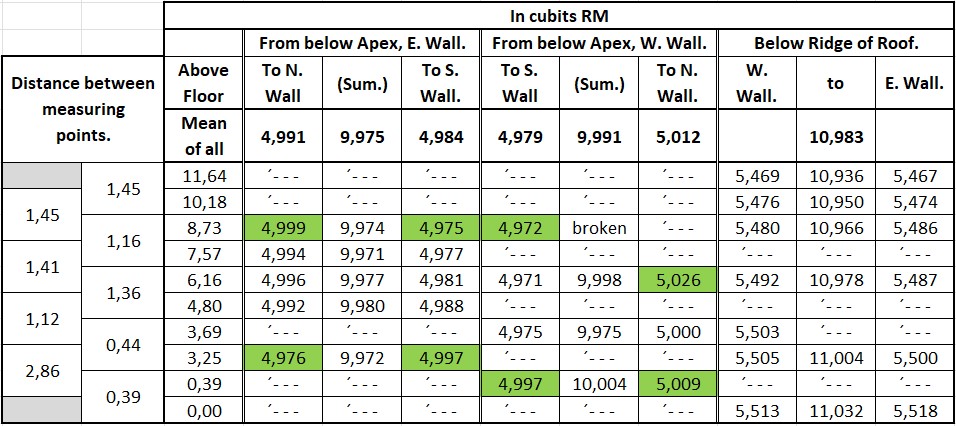
I have transferred Petrie´s table into an Excel sheet, so it is easier to see why Petrie focused on the deviations, underneath are the results in inches:
Underneath are the results in cubits:
As all the deviations are under +/- 0,1 cubits, the results have no influence on the coordinates.
In the next table are the distances calculated in cubits as well:
The green marked values will be inserted in the following drawings.
Furthermore you can read the vertical distances between the measuring points.
Petrie wished to show that the walls are not perfectly vertical:
Both the northern and southern walls seems to bend a bit against north the higher Petrie measured in the chamber.
The western wall seems to bend a bit against east and the eastern wall bend a bit towards west the higher Petrie measured in the chamber.
Section 5.8.8
It seems the row of the wall stones, the so-called courses, are more or less the same in all 4 walls. Piazzi Smyth made a drawing of the chamber in which you can see the courses as horizontal lines:Source: S21. "The Great Pyramid Its Secrets and Mysteries Revealed" by C. Piazzi Smyth. Fourth and much enlarged edition. New York, Bell Publishing Company, 1990 edition, reprint of the 1880 ed. published by W. Ibister, London under title: Our Inheritance in The Great Pyramid. Plate IX in the chapter "Corrigenda" in part lll.
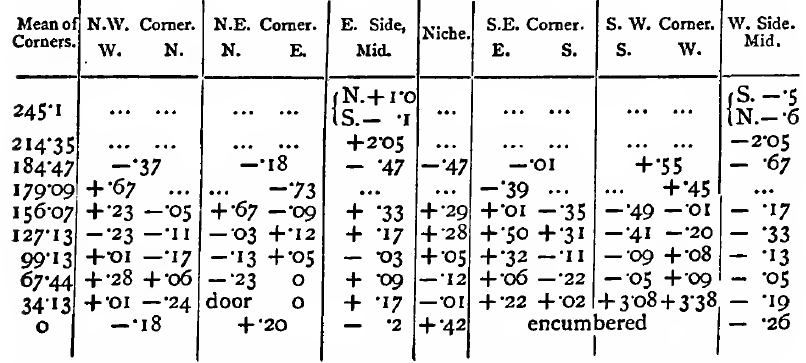
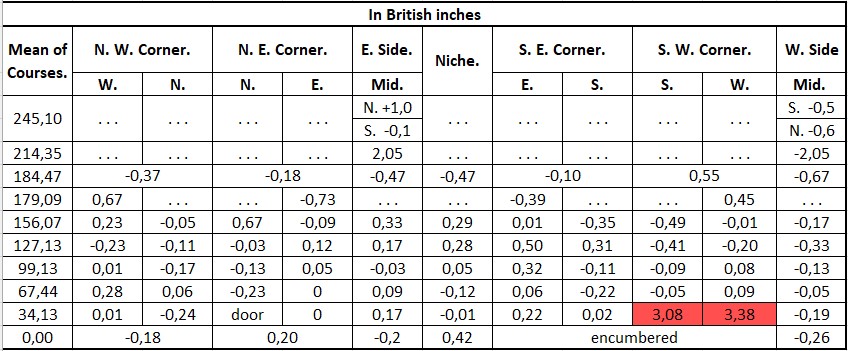
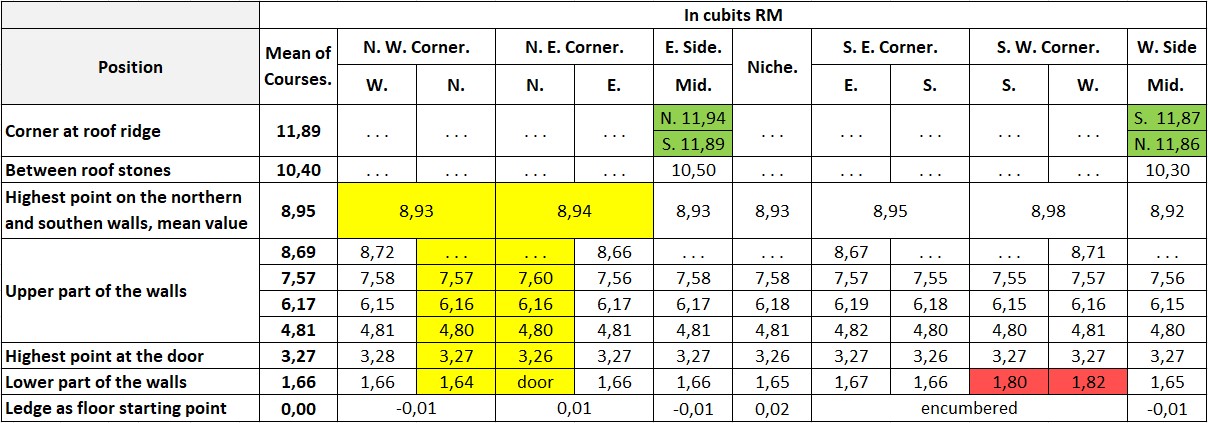
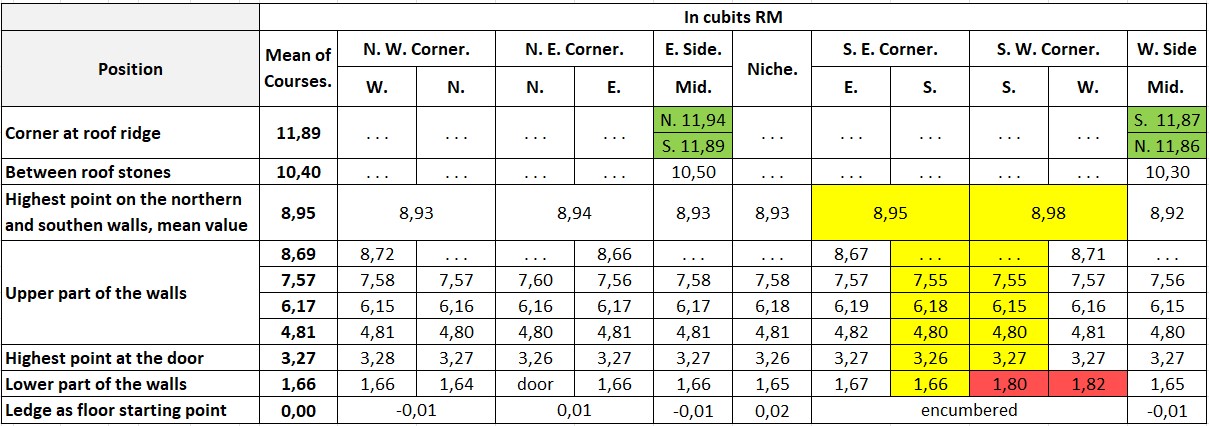
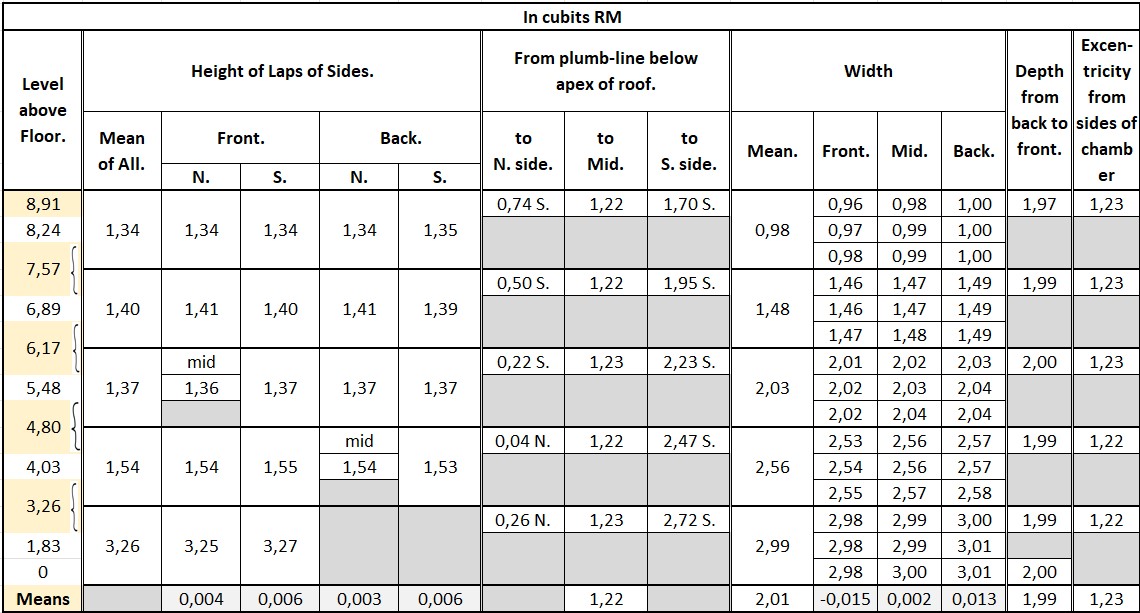
Flinders Petrie measured the corners of the courses:
Source: (S5orig-[S42]-P68-table)
The first column is the mean values of the corners in British inches. All the other columns contain deviations from the mean values in the first column.
There are three set of deviations which are remarkable:
The first set is mentioned at 245,1", which is the apex of the eastern and western walls. Petrie explained the deviations as a result of the sloping roof stones have fallen a little.
The second set is mentioned at 214,35" : "+2,05" on mid of the eastern wall and "-2,05" on the mid of the western wall. The mean deviation is zero.
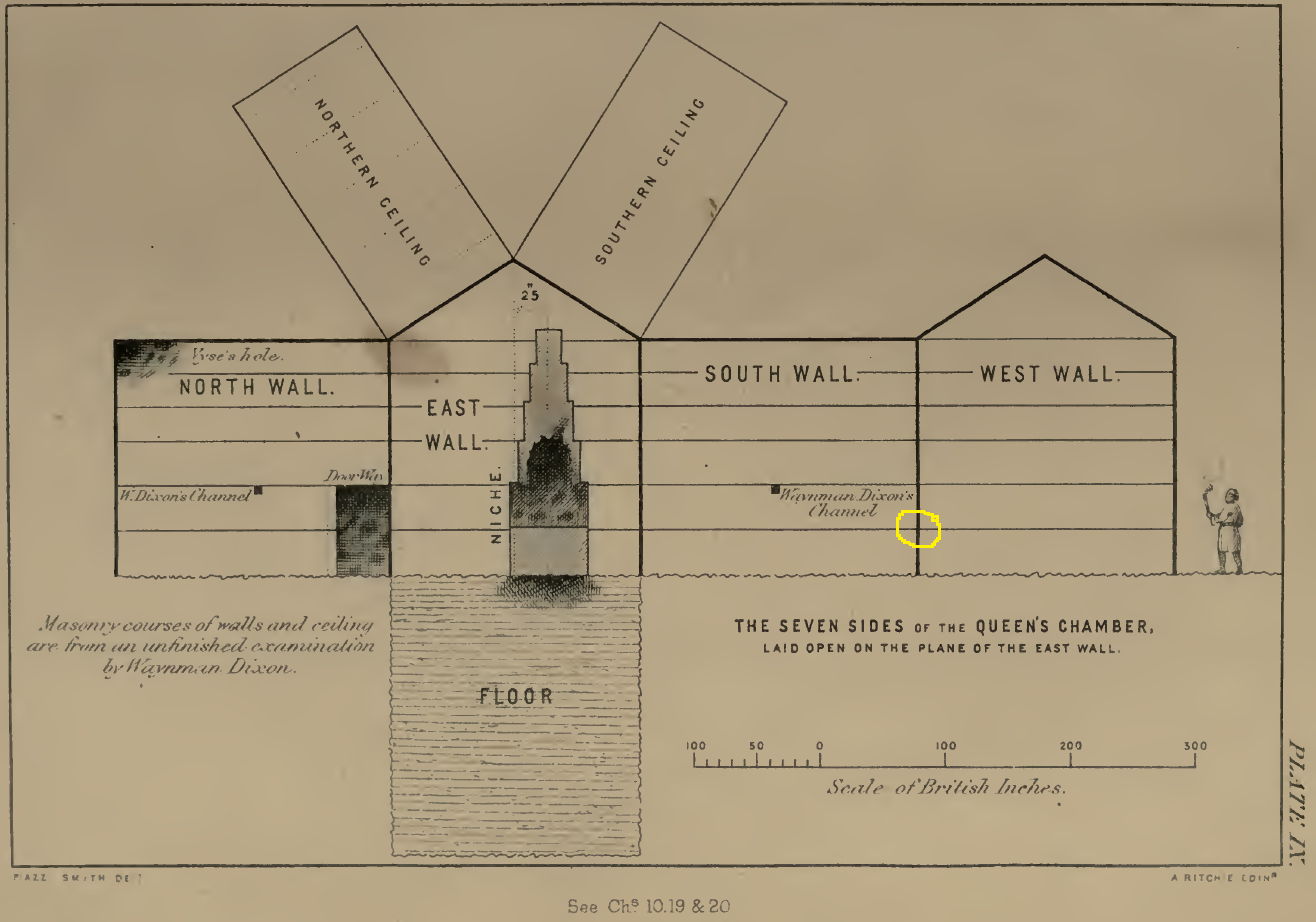
The third set is mentioned at 34,13" in the column "S. W. Corner," in the sub columns "S." and "W." with the values +3,08", and +3,38". These deviations cannot be explained in another way than a result of typing errors. My argument is based on maths and Smyth´s drawing:
1. Argument: The value 34,13" is a mean value, which means the mean value of the deviations in a row must be closed to zero, (except the course at 184,47" close to the sloping roof stones). The mean value of the deviations at 34,13" is +0,1" -0,24" +0" +0,17" -0,1" +0,22" +0,2" +3,08" +3,38" -0,19" = 0,64", which is not acceptable. If we take out the two values I suspect are a typing error , then we get
+0,1" -0,24" +0" +0,17" -0,1" +0,22" +0,2" -0,19" = 0,00"
2. Argument: The huge deviation in the 2nd course from the floor would have been detected by Smyth in 1880 and the drawing would clearly have shown other lines in the south-west corner, but the lines are not bended, they are straight. Please see the drawing hereunder with the yellow marking.
The conclusion is, we must disregard from the two deviations +3,08" and +3,38".
3,08" is 7,8 cms and 3,38" is 8,6 cms, it is impossible Smyth would have ignored such deviations.
Source: Source: S21. "The Great Pyramid Its Secrets and Mysteries Revealed" by C. Piazzi Smyth. Fourth and much enlarged edition. New York, Bell Publishing Company, 1990 edition, reprint of the 1880 ed. published by W. Ibister, London under title: Our Inheritance in The Great Pyramid. Plate IX in the chapter "Corrigenda" in part lll. Revised by me.
Section 5.8.9
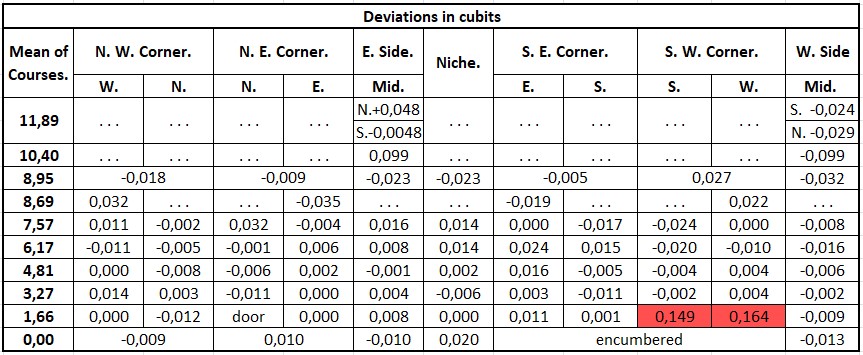
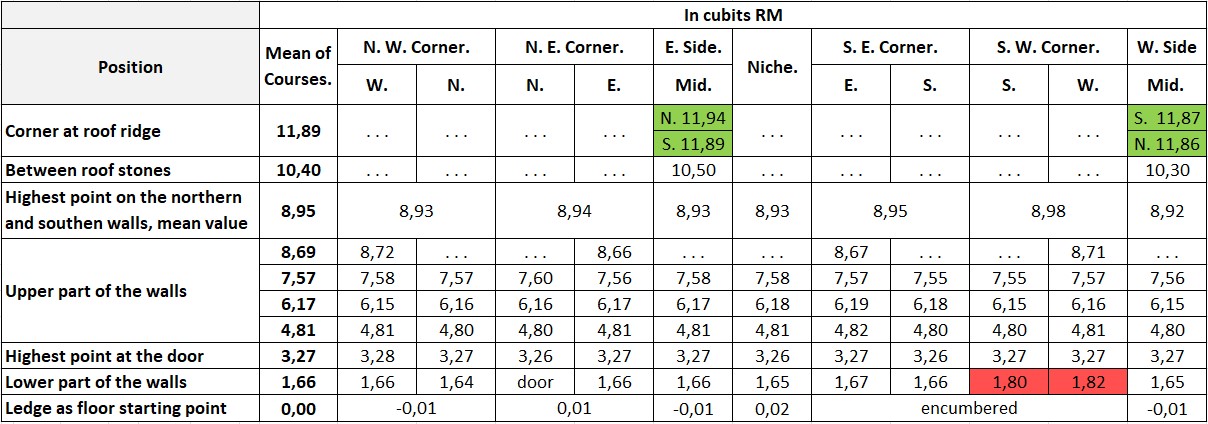
I have transferred the data from above table in section 5.8.8 and marked the two disregarded deviations with red:The next table is the same, but the deviations are in cubits:
The next table is also the same, but the measuring values are in cubits RM:
The green marked values will be inserted in the following drawings.
Calculation: The mean values are added to the deviations. For example, the mean value 11,89 has been added to 0,048, which gives 11,94.
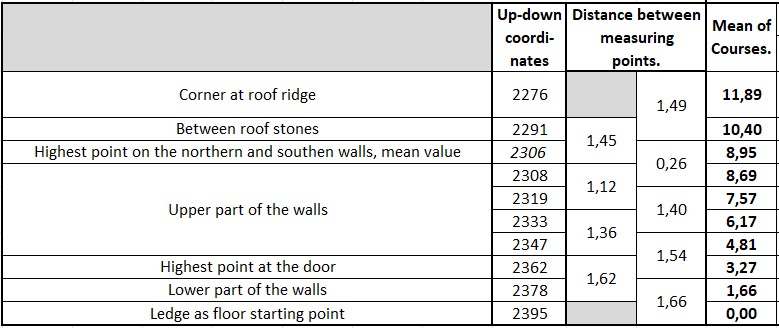
The up-down coordinates for the courses are:
Calculation: From the "floor" level the distances between the mean of courses x 10 has been subtracted from 2395, which is the floor level according to section 5.8.5.
Examples:
2395 - (1,66 x 10) = 2378
2395 - (3,27 x 10) = 2362
and so on..
The measures from above sections are in the following drawings.
Hereunder the eastern wall:
(The niche will be added in a later section.)
And the western wall:
Section 5.8.10
The holes in the northern and the southern walls leading to the two channels are not properly explained where they are exactly in the walls. The only information I have about the holes is from Petrie:"The N. channel is 8.6 high, and about 8 wide in the chamber wall, running horizontally for 76 inches, and then turning upwards. The S. channel is 8.8 high, and runs 80.0 to its turn upwards."
(S5orig-[S44]-P70-L38-40)
My comments:
Northern hole:
8,6" high = 0,417 cubits
8,0" wide = 0,388 cubits
Southern hole:
8,8" high = 0,427 cubits
Wide = ?
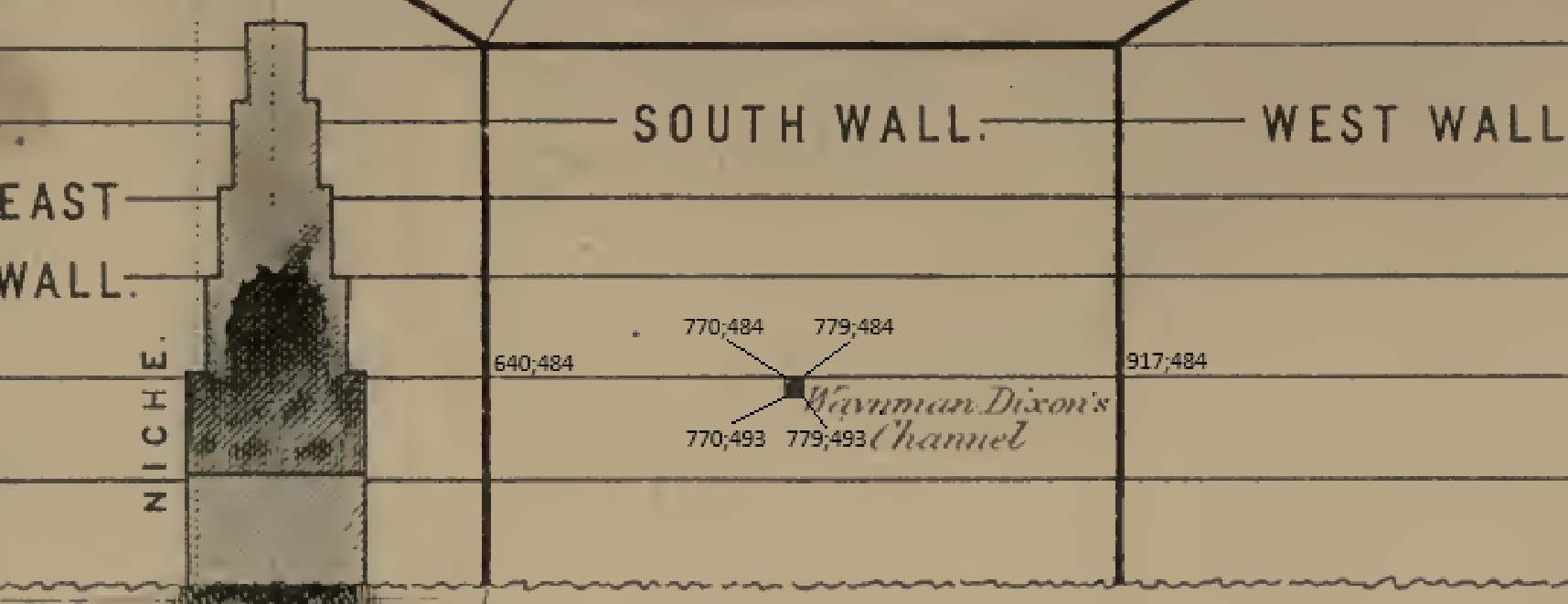
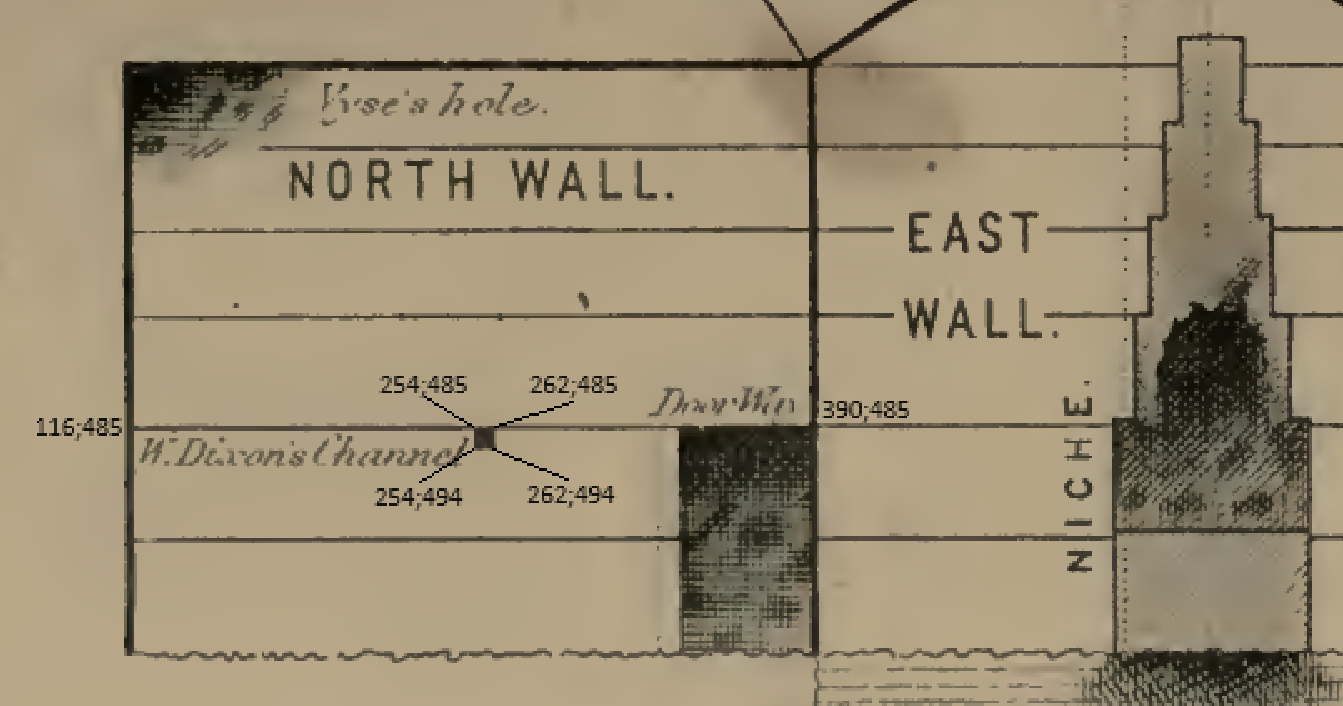
There is no hint where they are placed in the walls, but Smyth has made a drawing, so by measuring the relevant coordinates in the drawing, it is possible to calculate where the holes are located with a decent accuracy.
The relevant coordinates on the southern wall:
Source:
Source:
S21. "The Great Pyramid Its Secrets and Mysteries Revealed" by C. Piazzi Smyth. Fourth and much enlarged edition. New York, Bell Publishing Company, 1990 edition, reprint of the 1880 ed. published by W. Ibister, London under title: Our Inheritance in The Great Pyramid. Plate IX in the chapter "Corrigenda" in part lll. Revised by me.
The southern hole is a part of a stone in the 2nd course from the floor.
The joint between the 2nd and the 3rd course against the eastern wall is 640;484, which means 640
pixels(drw)
in the horizontal axis and 484
pixels(drw)
in the vertical axis.
The joint between the 2nd and the 3rd course against the western wall is 917;484, which means 917 pixels(drw) in the horizontal axis and 484 pixels(drw) in the vertical axis.
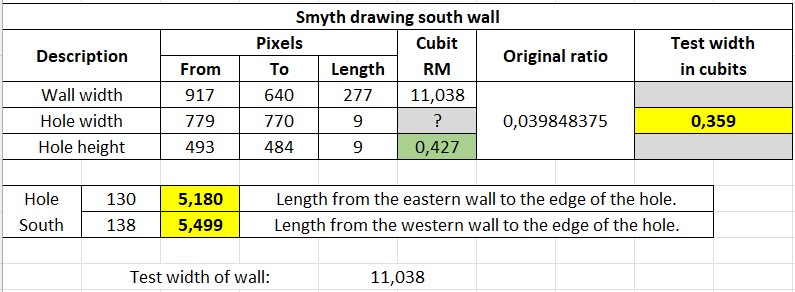
The distance between the western and eastern walls is 917 pixels(drw) - 640 pixels(drw) = 277 pixels(drw) . As the floor is rough and uneven we ignore the vertical result in this case.
We can use the 277
pixels(drw)
as a reference. We know from section 5.8.4, that the length of the southern wall at the floor is 227,61" = 11,038 cubits RM.
The ratio is therefore 11,038 cubits / 277 pixels(drw) = 0,039848375 cubits/pixel(drw).
From the eastern wall to the edge of the hole, the distance is 770 pixels
(drw)
- 640 pixels(drw)
= 130 pixels(drw), which is 5,2 cubits (rounded).
From the western wall to the edge of the hole, the distance is 917 pixels(drw)
- 779 pixels(drw)
= 138 pixels(drw), which is 5,5 cubits (rounded).
The width of the hole in the southern wall is 9 pixels(drw) x 0,039848375 cubits/pixel(drw) = 0,4 cubits (rounded).
The drawing of the northern wall is here:
Source:
Source:
S21. "The Great Pyramid Its Secrets and Mysteries Revealed" by C. Piazzi Smyth. Fourth and much enlarged edition. New York, Bell Publishing Company, 1990 edition, reprint of the 1880 ed. published by W. Ibister, London under title: Our Inheritance in The Great Pyramid. Plate IX in the chapter "Corrigenda" in part lll. Revised by me.
The northern hole is also a part of a stone in the 2nd course from the floor.
The joint between the 2nd and the 3rd course against the eastern wall is 390;485, which means 390 pixels in the horizontal axis and 485 pixels in the vertical axis.
The joint between the 2nd and the 3rd course against the western wall is 116;485, which means 116 pixels in the horizontal axis and 485 pixels in the vertical axis.
The distance between the western and eastern walls is 390 pixels(drw) - 116 pixels(drw) = 274 pixels(drw).
We can use the 274 pixels(drw) as a reference. We know from section 5.8.4, that the length of the northern wall at the floor is 227,41" = 11,029 cubits RM.
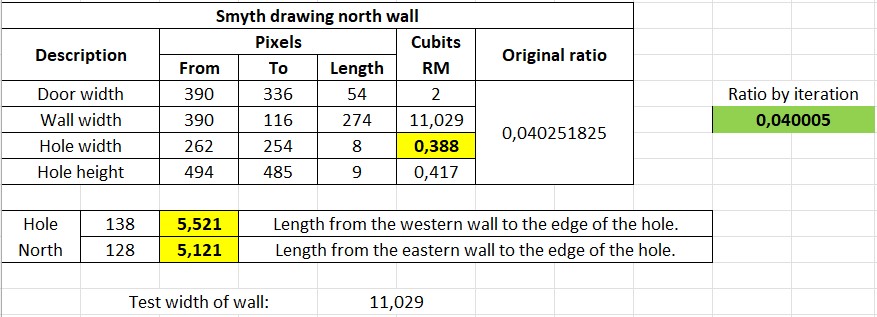
The original ratio is 11,038 cubits RM / 274 pixels(drw) = 0,04025185 cubits/pixel(drw). As the drawing is not crystal clear, it was necessary to adjust the original ratio by a iteration to make sure the calculated width of the northern wall was exactly or close to the length measured by Petrie.
The result of the iteration is a ration of 0,040005; which is a ratio deviation of 0,00024685.
From the eastern wall to the edge of the hole, the distance is 390 pixels
(drw)
- 262 pixels(drw)
= 128 pixels(drw), which is 5,1 cubits (rounded).
From the western wall to the edge of the hole, the distance is 254 pixels(drw)
- 116 pixels(drw)
= 138 pixels(drw), which is 5,5 cubits (rounded).
Section 5.8.11
The upper edge of both the northern and southern holes follow the upper edge of the of the 2nd course of stones, which again follow the upper edge of the door. The up/down coordinate for this edge is 2363 according to section 5.8.5. The floor footing up/down coordinate is 2395 also according to section 5.8.5.
The height of the northern hole is 0,417 cubits RM according to section 5.8.10, which is 4 pixels.
The height of the Southern hole is 0,427 cubits RM according to section 5.8.10, which is 4 pixels as well.
So, for each holes the up/down coordinate for the lower edge is 2363 pixels + 4 pixels = 2367.
The height between the lower edge of the holes and the floor footing is (2395 - 2367) / 10 = 2,8 cubits, equal to 3,96 remens (close to 4 remens).
Source: Pinterest, the reference is drhawass.com, but the URL is no longer active.
Section 5.8.12
The holes were unknown until the year 1872 by Mr. Waynman Dixon.
(S5orig-[S44]-P70-L36)
The holes were hidden by a thin wall in the original stone; it is possible to see a remnant in the northern hole.
Source: Pinterest, the reference is drhawass.com, but the URL is no longer active.
Section 5.8.13
The channels behind the holes, which should not have been exposed, will be examined later. I am still collecting data...Section 5.8.14
The niche is described very well by Petrie:
"The niche in the eastern wall of this chamber, from its supposed connection with a standard of measure, was very closely examined. Its original depth back was certainly only 41 inches at every part from the bottom upwards. The surface that might be supposed to belong to the side of a deeper part, is only that of a joint of masonry, one stone of which has been broken up and removed ; this is evident as there is mortar sticking to it, and as it is pick-dressed, quite different to the fine surfaces of the niche sides ; beside this, it is not flush with the side, or any of the overlappings of the niche ; and moreover, all down the niche sides are the traces of the edge of the back, at 41 from the front, where it has been broken away.The general form of the niche was a recess 41 inches (2 cubits) deep back ; 62 inches (3 cubits) wide at base, and diminishing its width by four successive overlappings of the sides (at each wall course), each of 1/4 cubit wide, until at 156 high it was only 20 (1 cubit) wide, and was finally roofed across at 184 high. Thus, of the 3 cubits width of the base, one cubit was absorbed on each side by the overlappings, leaving one cubit width at the top. This cubit is the regular cubit of 20.6 inches, and there is no evidence of a cubit of 25 inches here. The exact dimensions of every part are as follow, giving the mean dimensions, and the variations of each part, + or —, from the mean. All corrected for the salt exudation on the two lower laps, as estimated at each point ; there is no salt on the upper three laps :"
(S5orig-[S43]-P69-L31-40 and P70-L1-11)
Petrie added these measurements:
Source:
(S5orig-[S43]-P70-table)
I have transferred the data into Excel:
The unit is British inches.
And the same with absolute values in cubits:
Section 5.8.15
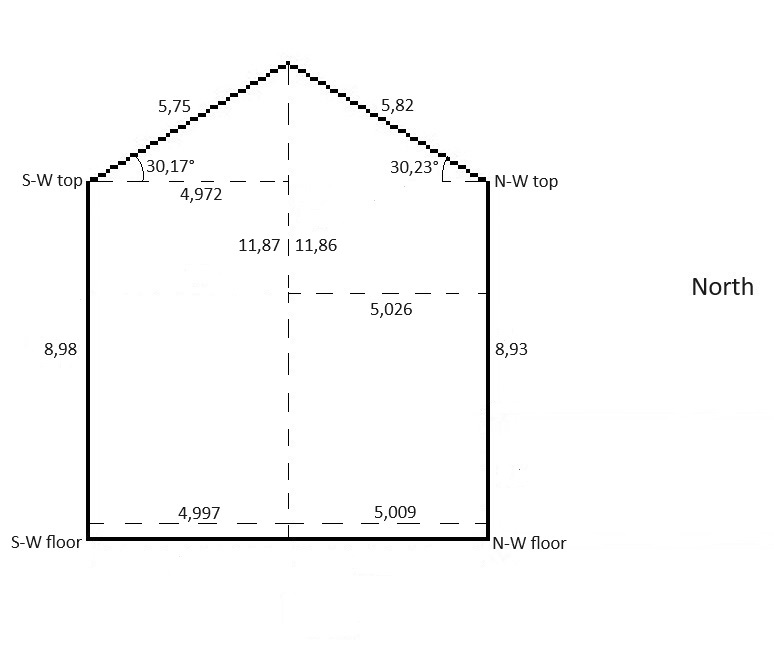
Resume.The overall E-W and N-S lengths for the MASTER drawing in the Queens chamber are:
Width: 9,98 cubits RM (average) wide, which equals 100 pixels (acc. to section 5.8.4).
Length: 10,98 cubits RM (average) long * , which equals 110 pixels (acc. to section 5.8.4).
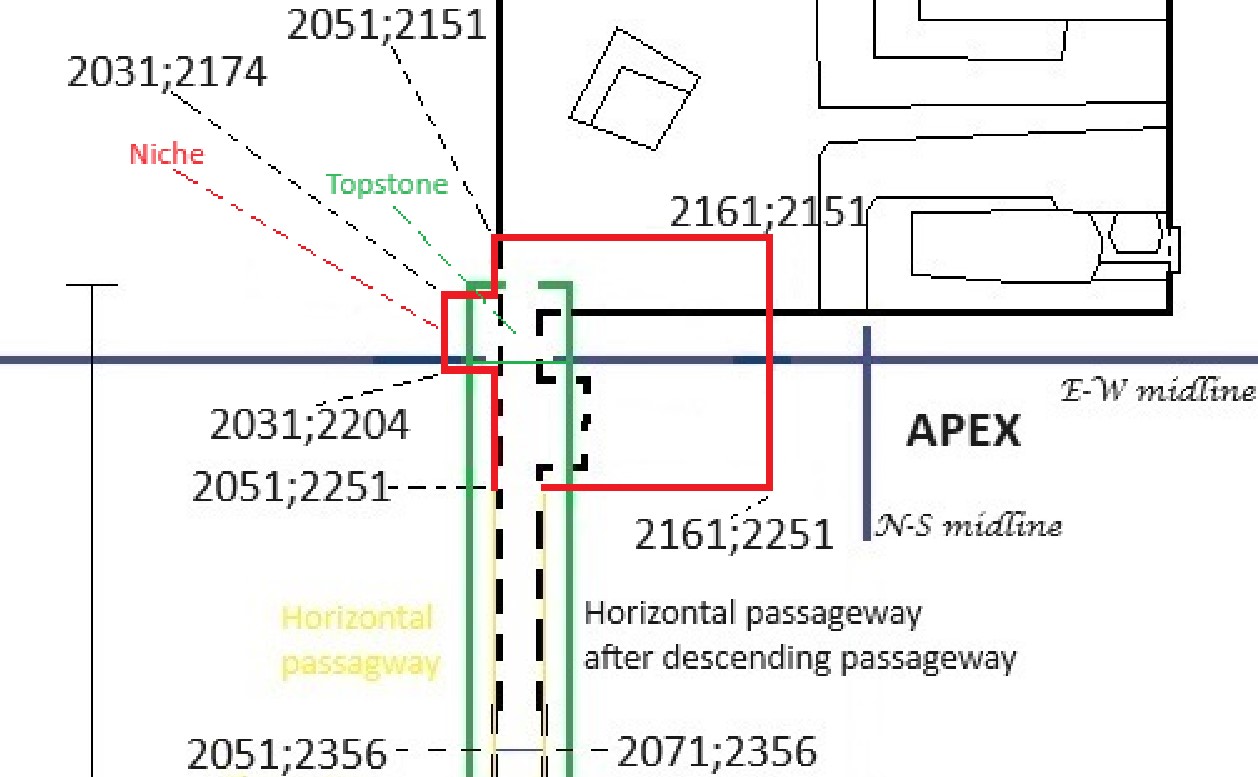
The Niche at floor level:
Width: 2,99 cubits RM (average) wide, which equals 30 pixels (acc. to section 5.8.14).
Length from Apex of roof to northern edge: 0,26 cubits RM, which equals 3 pixels (acc. to section 5.8.14).
Length from Apex of roof to southern edge: 2,72 cubits RM, which equals 27 pixels (acc. to section 5.8.14).
Length from Apex of roof to mid of the Niche: 1,23 cubits RM, which equals 12 pixels (acc. to section 5.8.14).
The depth of the Niche: 1,99 cubits RM, which equals 20 pixels (acc. to section 5.8.14).
The Apex of the roof has the N-S coordinate: 2201 (acc. to section 5.8.5).
The door opening has these E-W;N-S coordinates:
At eastern edge: 2051;2251
At western edge: 2071;2251
The coordinate of the south - east corner of the chamber: 2051;(2251-100) = 2051;2151
The coordinate of the south - west corner of the chamber: (2051+110);2151 = 2161;2151
The coordinate of the north - west corner of the chamber: 2161;(2151+100) = 2161;2251
The coordinate of the north - east corner of the chamber: (2161-110);2251 = 2051;2251
Northern edge of the Niche is 0,26 cubits RM or 3 pixels north of the APEX of the pyramid which is at 2200 pixels.
The coordinate of the northern edge of the Niche on the eastern wall: 2051;(2201+3) = 2051;2204
The coordinate of the southern edge of the Niche on the eastern wall: 2051;(2204-30) = 2051;2174
The coordinate of the northern edge of the Niche in the eastern wall: (2051-20);2204 = 2031;2204
The coordinate of the southern edge of the Niche in the eastern wall: (2051-20);2174 = 2031;2174
Section 5.8.16
Here is a top view. The Queens chamber has red lines:And a bit closer:
It is interesting, the Niche is more or less on the same N-S location as the topstone in the Grand Gallery.
Section 5.8.17
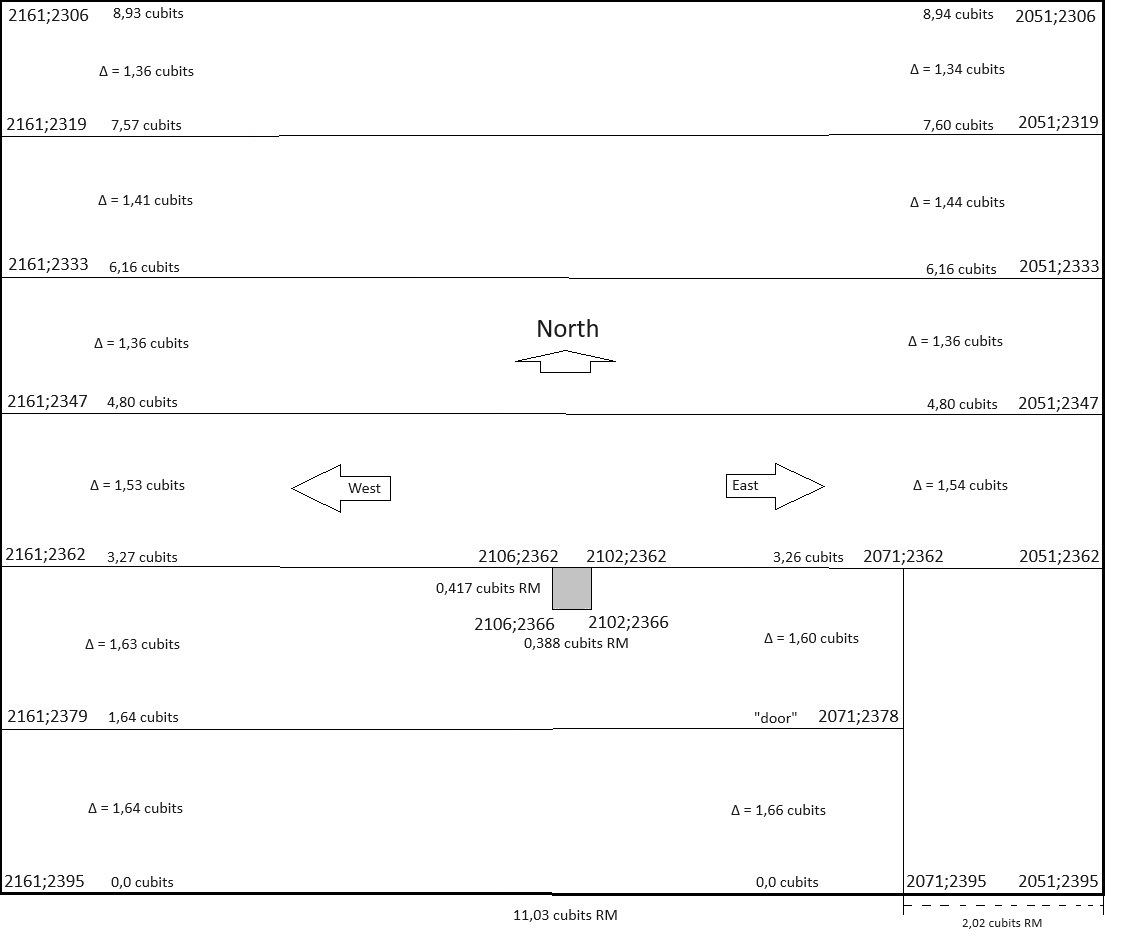
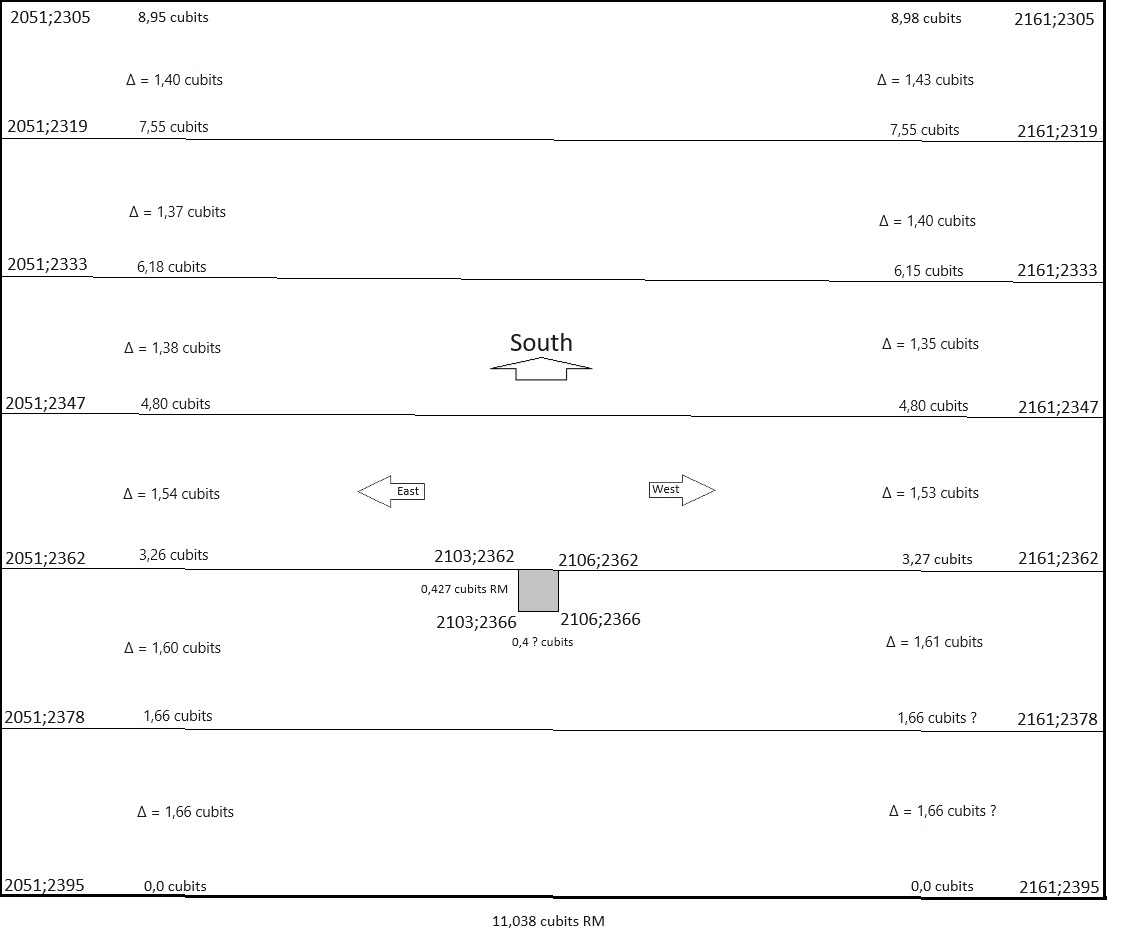
Drawing of the northern wall in the Queens chamber, based on the values marked yellow in the table below, same as the table in section 5.8.9 :The drawing of the northern wall in the Queens chamber:
The coordinates are E-W;Up-Down.
Please note that the above drawing has east on the right side and west on the left side, as if you are standing in the chamber with your back to the south and looking north.The total height of the courses are from the table in section 5.8.9
The floor has the mean value 0,0 cubits in the drawing.
The delta sign represents the difference in the heights between two courses.
The sizes of the hole measured by Petrie are mentioned in section 5.8.10
The position of the hole in the east-west and up-down directions are calculated from the drawing made by Smyth in section 5.8.10, from source S21.
The calculation method of the courses is the usual, example:
The floor corner at the eastern wall is 2051;2395 as stated in the sections 5.8.16 (east-west) and 5.7.25 (up-down). The length of the northern wall is 11,03 cubits RM. The floor corner at the western wall is then (2051 +110 ; 2395) = 2161;2395. The east-west coordinate 2161 is verified in the drawing in section 5.8.16
All the other coordinates are calculated the same way.
Section 5.8.18
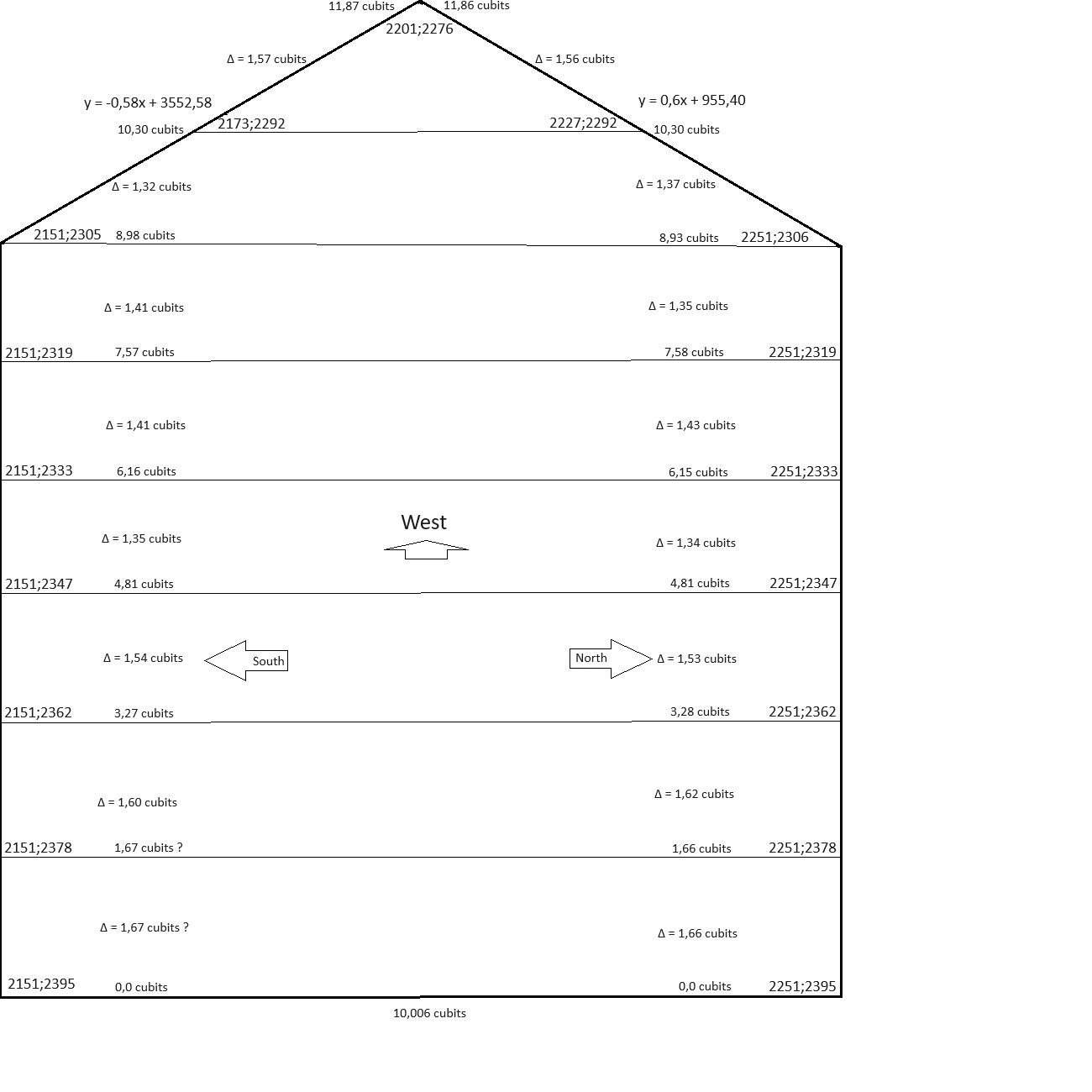
Drawing of the western wall in the Queens chamber, based on the values marked yellow in the table below , same as the table in section 5.8.9:
The drawing of the western wall in the Queens chamber:
The coordinates are N-S;Up-Down.
Please note that the above drawing has south on the right side and north on the left side, as if you are standing in the chamber with your back to the east and looking west.The total height of the courses are from the table in section 5.8.9
The floor has the mean value 0,0 cubits in the drawing.
The delta sign represents the difference in the heights between two courses.
The calculation method of the courses is the usual, example:
The floor corner at the northern wall is 2251;2395 as stated in the sections 5.8.16 (north-south) and 5.7.25 (up-down). The length of the western wall is 10,006 cubits RM. The floor corner at the southern wall is then (2251 -100 ; 2395) = 2151;2395. The east-west coordinate 2151 is verified in the drawing in section 5.8.16
All the other coordinates are calculated the same way.
Section 5.8.19
Drawing of the southern wall in the Queens chamber, based on the values marked yellow in the table below , same as the table in section 5.8.9:The drawing of the southern wall in the Queens chamber:
The coordinates are E-W;Up-Down.
Please note that the above drawing has east on the right side and west on the left side, as if you are standing in the chamber with your back to the north and looking south.The total height of the courses are from the table in section 5.8.9
The floor has the mean value 0,0 cubits in the drawing.
The delta sign represents the difference in the heights between two courses.
The calculation method of the courses is the usual, example:
The floor corner at the eastern wall is 2051;2395 as stated in the sections 5.8.16 (east-west) and 5.7.25 (up-down). The length of the western wall is 11,0,38 cubits RM. The floor corner at the western wall is then (2051 -110 ; 2395) = 2161;2395. The east-west coordinate 2161 is verified in the drawing in section 5.8.16
All the other coordinates are calculated the same way.
Section 5.8.20
Drawing of the eastern wall in the Queens chamber, based on the values marked yellow in the table below , same as the table in section 5.8.9:And the table in section 5.8.14 showing the measures of the Niche:
The coordinates are N-S;Up-Down.
Please note that the above drawing has north on the right side and south on the left side, as if you are standing in the chamber with your back to the west and looking east.The width of the eastern wall is 9,973 cubits RM according to section 5.8.9.
The northern end is in total: (3,25 + 1,54 + 1,36 + 1,41 + 1,34) cubits = 8,90 cubits.
The southern end is in total: (3,27 + 1,55 + 1,37 + 1,40 + 1,34) cubits = 8,93 cubits.
The difference is 0,03 cubits which is lower than my accuracy of +/- 0,1 cubits; this results in the same up-down coordinates on the top of the niche, but you can see it in the much more detailed drawing.
The mid of the chamber has the N-S coordinate 2201.
The floor is defined as 0,0 cubits in the chamber and has the Up-Down coordinate 2395.
Section 5.8.21
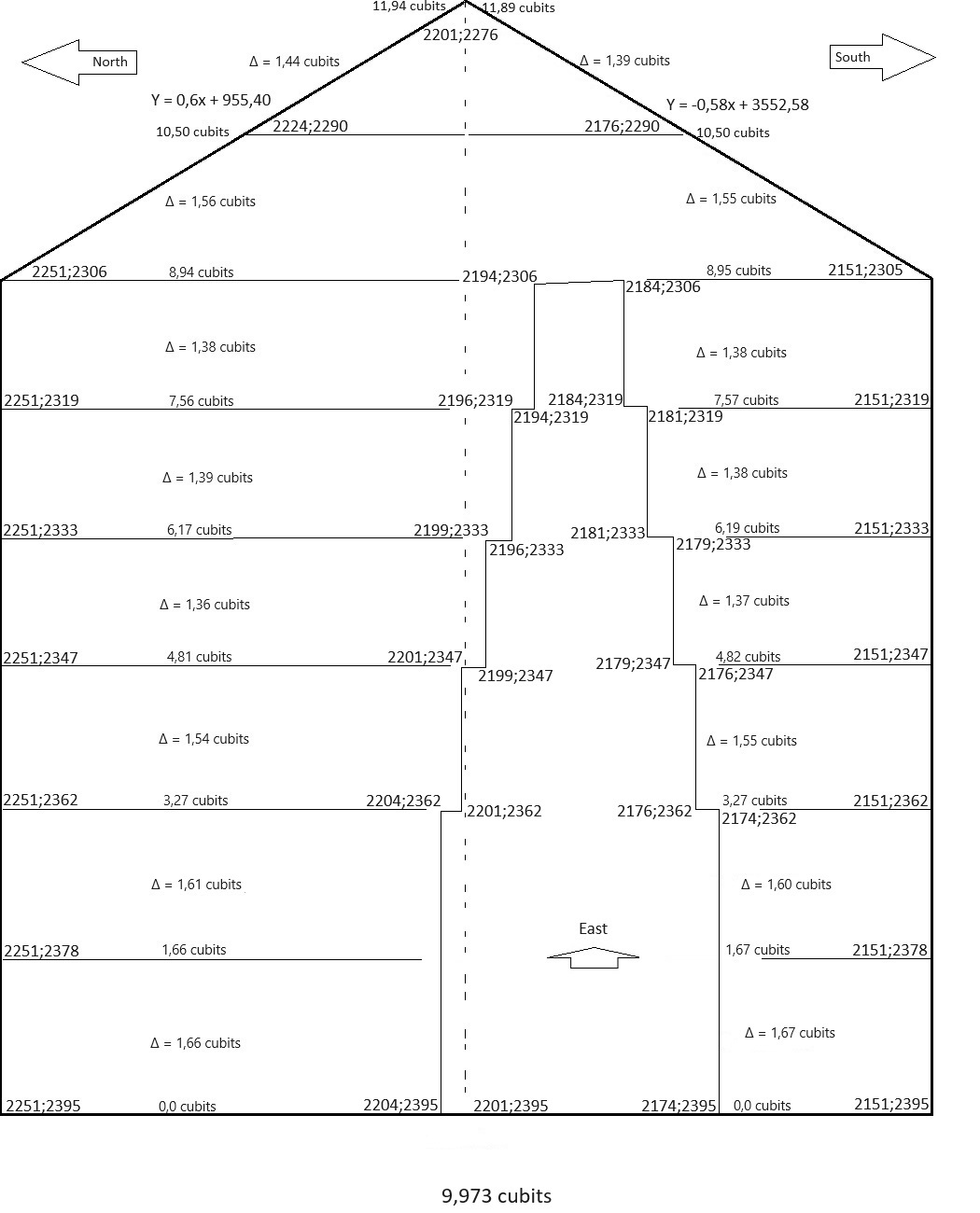
The calculation method of the coordinates of the courses is:
The N-S; Up-Down coordinates of the floor corners at the southern wall is
2151;2395
northern wall is 2251;2395 as stated in the sections 5.8.5.
The corners of the courses from the floor to the roof on the northern side are:
2251;2395
(2251;2395-17) = 2251;2378
(2251;2395-33) = 2251;2362
(2251;2395-48) = 2251;2347
(2251;2395-62) = 2251;2333
(2251;2395-76) = 2251;2319
(2251;2395-89) = 2251;2306
(2251;2395-105) = 2251;2290
((2290-955,40)/0,6;2395-105) = 2224;2290
((2276-955,40)/0,6;2395-119) = 2201;2276
The corners of the courses from the floor to the roof on the southern side are:
2151;2395
(2151;2395-17) = 2151;2378
(2151;2395-33) = 2151;2362
(2151;2395-48) = 2151;2347
(2151;2395-62) = 2151;2333
(2151;2395-76) = 2151;2319
(2151;2395-90) = 2151;2305
((2290-3552,58)/(-0,58);2395-105) = 2176;2290
((2276-3552,58)/(-0,58);2395-119) = 2201;2276
Section 5.8.22
The calculation method of the coordinates of the corners in the niche is based on the mid of the chamber at floor: 2201;2395
The corners of the corners from the floor to the top of the niche on the northern side are:(2201+3;2395) = 2204;2395
(2201+3;2395-33) = 2204;2362
(2201+0,4;2395-33) = 2201;2362
(2201+0,4;2395-48) = 2201;2347
(2201-2;2395-48) = 2199;2347
(2201-2;2395-62) = 2199;2333
(2201-5;2395-62) = 2196;2333
(2201-5;2395-76) = 2196;2319
(2201-7;2395-76) = 2194;2319
(2201-7;2395-89) = 2194;2306
The corners of the corners from the floor to the top of the niche on the southern side are:
(2201-27;2395) = 2174;2395
(2201-27;2395-33) = 2174;2362
(2201-25;2395-33) = 2176;2362
(2201-25;2395-48) = 2176;2347
(2201-22;2395-48) = 2179;2347
(2201-22;2395-62) = 2179;2333
(2201-20;2395-62) = 2181;2333
(2201-20;2395-76) = 2181;2319
(2201-17;2395-76) = 2184;2319
(2201-17;2395-89) = 2184;2306
Section 5.8.23
This section is for internal use only and is about the special coordinates for the detailed drawing in section 5.8.20.drawing format: Width 1050 pixels x height 1300 pixels.
1 pixel is equal to 1/100 cubits.
1 cubits is equal to 100 pixels.
Mid line with N-S coordinate 2201 is 498 pixels according to drawing in section 5.8.9.
Floor level is defined as 1194 pixels as the height of the chamber is 11,94 cubits.
N-S;Up-Down coordinates in the northern part of the niche in the detailed drawing starting at floor:
Floor: [498-26;1194] = [472;1194]
1. Level, northern corner: [498-26;1194-325] = [472;869]
1. Level, southern corner : [498-4;869] = [494;869]
2. Level, northern corner : [494;869-154] = [494;715]
2. Level, southern corner : [498+22;715] = [520;715]
3. Level, northern corner : [520;715-154] = [520;579]
3. Level, southern corner : [498+50;579] = [548;579]
4. Level, northern corner : [548;579-141] = [548;438]
4. Level, southern corner : [498+74;438] = [572;438]
5. Level, northern corner : [572;438-134] = [572;304]
N-S;Up-Down coordinates in the southern part of the niche in the detailed drawing starting at floor:
Floor: [498+272;1194] = [770;1194]
1. Level, southern corner : [770;1194-327] = [770;867]
1. Level, northern corner : [498+247;867] = [745;867]
2. Level, southern corner : [745;867-155] = [745;712]
2. Level, northern corner : [498+223;712] = [721;712]
3. Level, southern corner : [721;712-137] = [721;575]
3. Level, northern corner : [498+195;575] = [693;575]
4. Level, southern corner : [693;575-140] = [693;435]
4. Level, northern corner : [498+170;435] = [668;435]
5. Level, southern corner : [668;435-134] = [668;300]