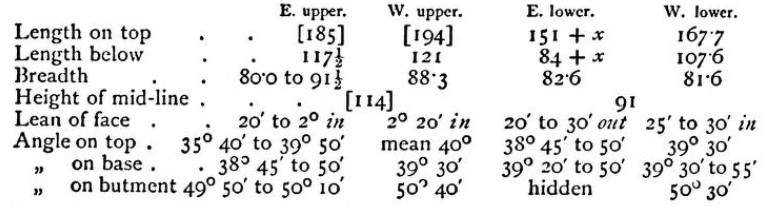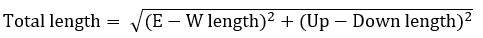The Entrance
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910
Section 5.1.1
This old picture tells us a lot. First of all we can see the outer casing stones are more or less missing. On the right side at the basis you can see two originally placed casing stones. Under the two casing stones and to the left you can see the original pavement stones. The internal lime stones, which are the main part of the masonry, are therefore visible. Originally there were approximately 210 courses of lime stones, but nowadays there are only 201.
There are 3 "entries", it is easy to see two of them in the above picture.
(All entries were originally covered by the casing stones.)
The big entry in the top of the picture, the so called chevron, is a fake entry, its basis hit the 22nd course. Behind the huge stones there is a passageway.
On the right side there is a hole made by the Arab caliph Abdullah al-Mamun in AD 820, which is the 2nd entrance (at 7th course).
The 3rd entrance is visible in the picture of an old drawing of this "fake" entrance (chevron) underneath at the 19th course just under the "fake" entrance.
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910
Section 5.1.2
Underneath is a picture of a part of the drawing of the 3 entrances made by Edwards, so you can see how they are located:
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910
The drawing indicates there had been more chevrons in front of the existing, which could be correct as there are angled stones left, which could have supported the missing chevrons.
Source : www.worldhistory.org/image/6192/entrance-passage-great-pyramid-of-giza/ (edited by me)
Flinders Petrie mentioned:
"The sloping blocks over the entrance to the Pyramid, and the space below them, were examined (partly by means of a ladder), and measured ; but the details are not worth producing here, as the work of them is so rough. The large blocks are as follows, in general size : —The measures in brackets are deduced from the angles and other measures. These blocks are much like a slice of the side of a casing stone in their angle ; but their breadth and length are about half as large again as any of the casing stones. Their mean angle from 12 measures is 50° 28' ± 5'. The thickness of these blocks is only 33 inches, and there are no others exactly behind them, as I could see the horizontal joints of the stones running on behind them for some inches. On the faces of these blocks are many traces of the mortaring which joined to the sloping blocks next in front of them. These were placed some 70 inches lower at the top, and were not so deep vertically. By the fragment left on the E. side, the faces of these blocks were vertical. In front of these came the third pair, similar, but leaning some 7 1/2 ° or 8 ° inwards on the face, judging by a remaining fragment. Probably a fourth and fifth pair were also placed here (see PI. ix.) ; and the abutment of the fifth pair shows an angle of 70 1/2° or 73° in place of 50°. The successive lowering of the tops, leaning the faces in, and flattening the angle of slope of the stones as they approach the outside, being apparently to prevent their coming too close to the casing."
(S5orig-[S34]-P53-L11-39)
My comments:
33 inches = 1,60 cubits RM
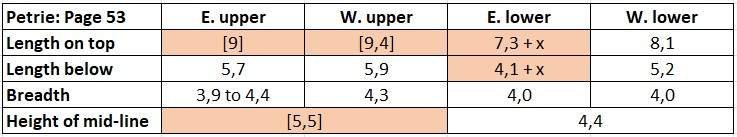
I have copied the above table to an Excel sheet:
The lengths and breadths are in inches.
Hereunder the inches are converted to cubits, those in brackets, and cubits RM, those without brackets,
The marked fields are not automatically accepted for further data processing.
Calculation method: Inches/20,62 = Cubits
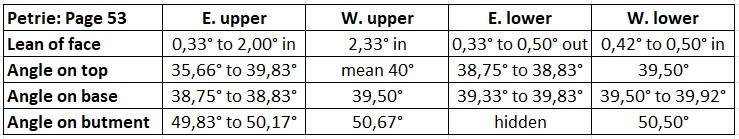
Here are the angles with decimals:
Calculation method: dd° mm´ = dd° + mm´/60 = dd,xx°
Section 5.1.3
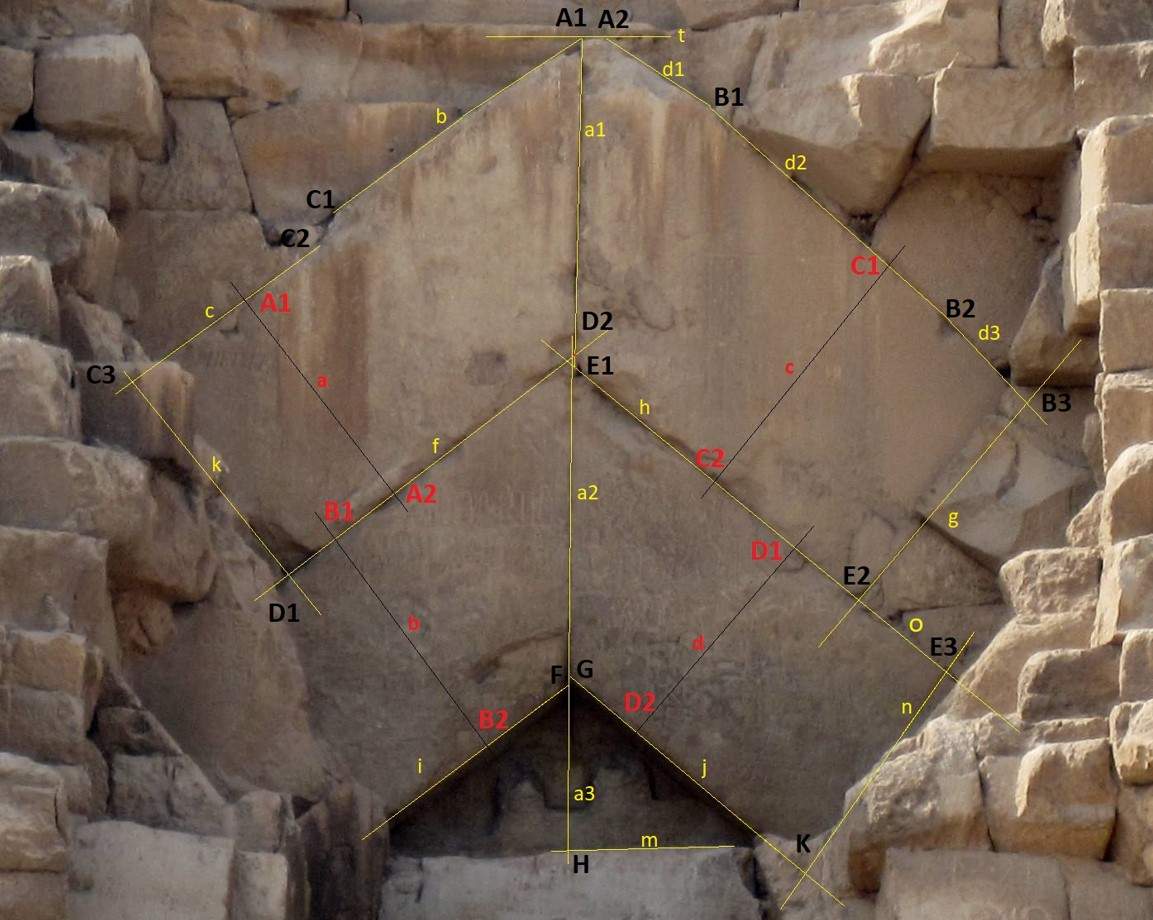
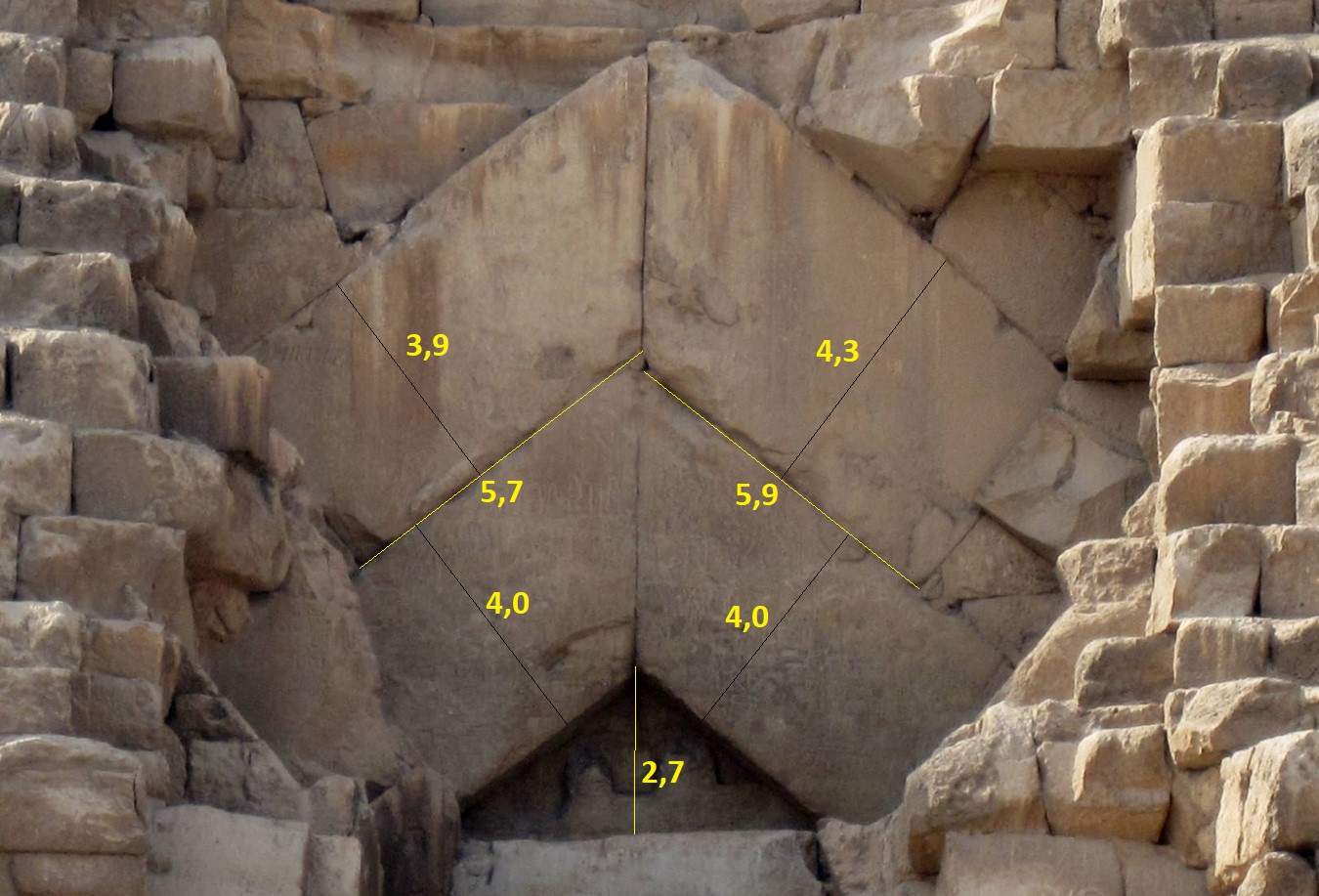
I have analyzed the measurements of the two pairs of angled stones, also called a chevron as it looks like the rank insignia of a Corporal in the US Army.
Here it is:It is not crystal clear where Petries above mentioned results were measured and we also need to verify the data.
The yellow lines follow the surfaces of the stones and the black lines are helping lines.
As you can see the upper part of the chevron are roughly made, so I need more lines to each stone.
Hereunder I have tagged the lines with small letters/small numbers and certain points with capital letters/big numbers:Source : https://www.worldhistory.org/image/6192/entrance-passage-great-pyramid-of-giza/
The yellow lines "a1" and "a2" are the midlines of the chevron.
The crosspoints "F" and "G" are the lowest points in the midline of the chevron.
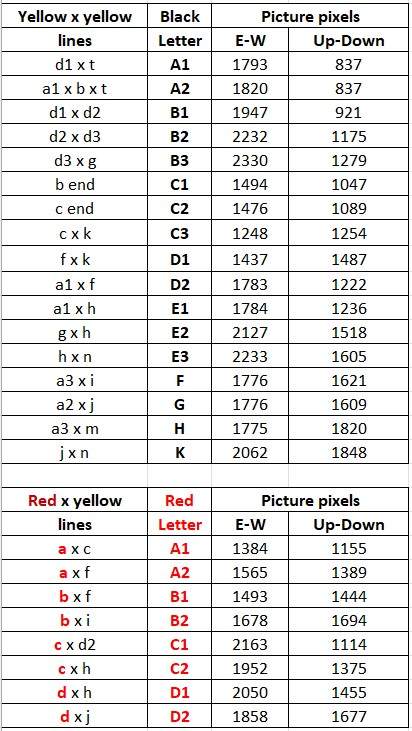
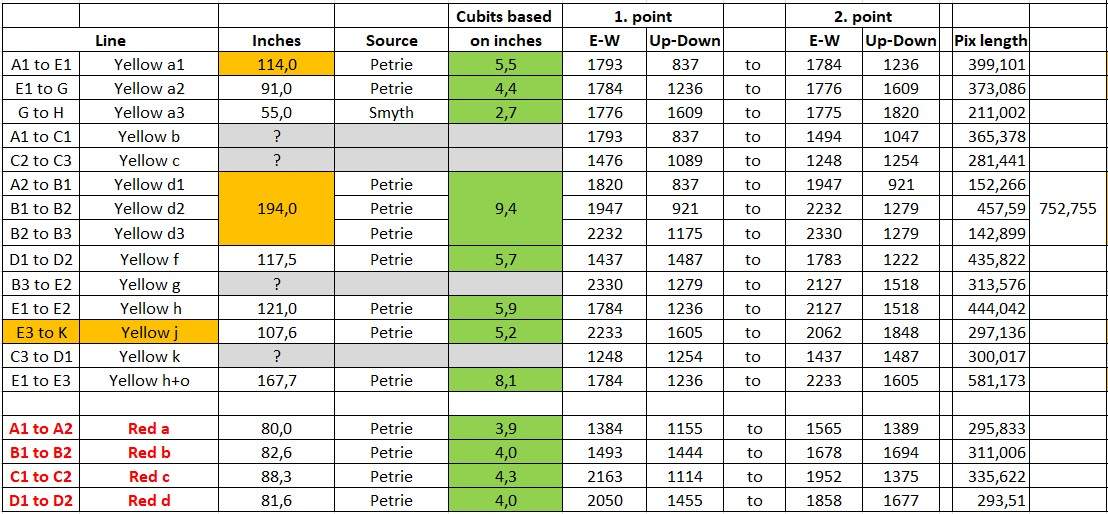
Hereunder are two tables with the positions in pixels of the points:
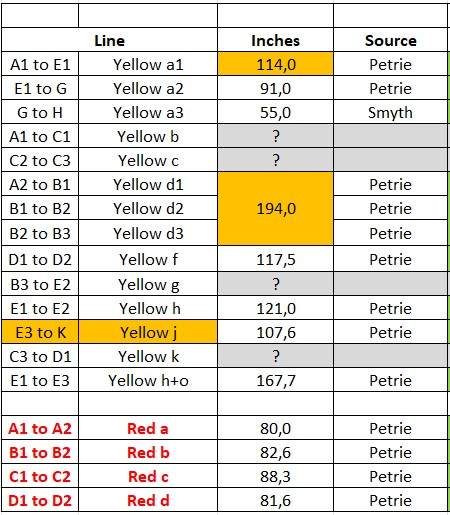
From Petries overview above we know following:
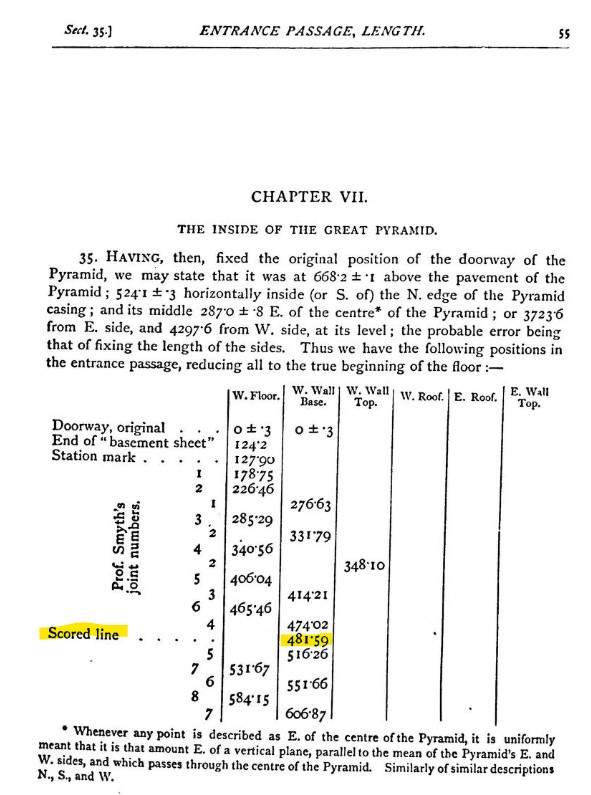
From cross point G to H Smyth is mentioned. The reference is here:
S.20. Life And Work at The Great Pyramid, during the months of January, February, March and April, A.D. 1865 by C. Piazzi Smyth, Vol. II, page 38 (part of the table) line 19.
The orange and grey markings indicate either the measurements are not complete or the values are calculated, so we must keep an eye on them in the following overviews.
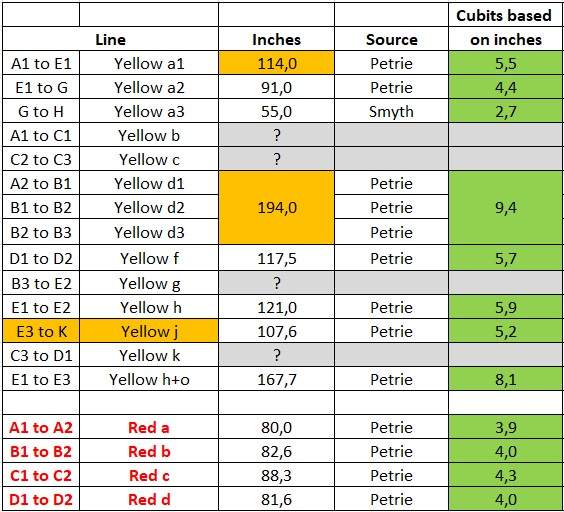
Hereunder I calculated the cubits based on the inches:
The next overview shows the positions of the points and the lengths of the lines are calculated in pixels:
Calculation method:
In the last overview we can see the verification of the data:
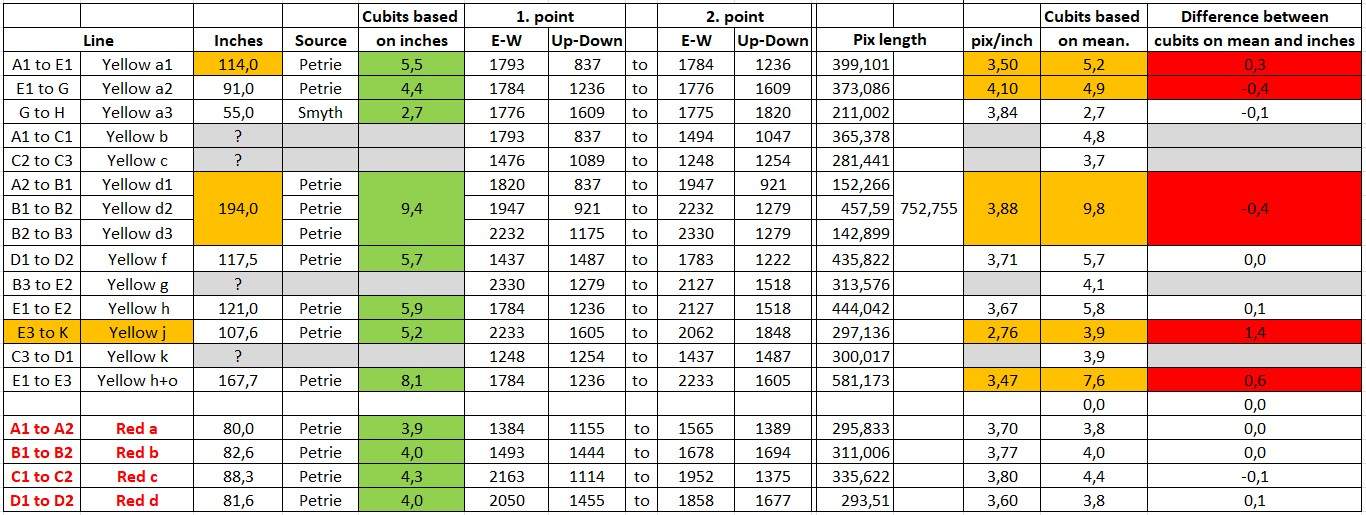
The mean value of the accepted data and other values of the pixels/inches values are here:
All differences over +/- 0,1 cubits are discarded.
It is not surprising the lines "Yellow a1", "Yellow d1,d2,d3" and "Yellow j" cannot be verified.
However; the line "Yellow a2", which was measures to 91" by Petrie is a surprise. It is the first time I cannot validate his measurement.
The line h+o is also discarded, but I must admit it could be my "Line n", which is wrong. it was nearly impossible to draw it as the angled stone is partly covered be casing stones.
Here are the validated measures in cubits RM:
Source : https://www.worldhistory.org/image/6192/entrance-passage-great-pyramid-of-giza/ (lines and measures are added by me)
Section 5.1.4
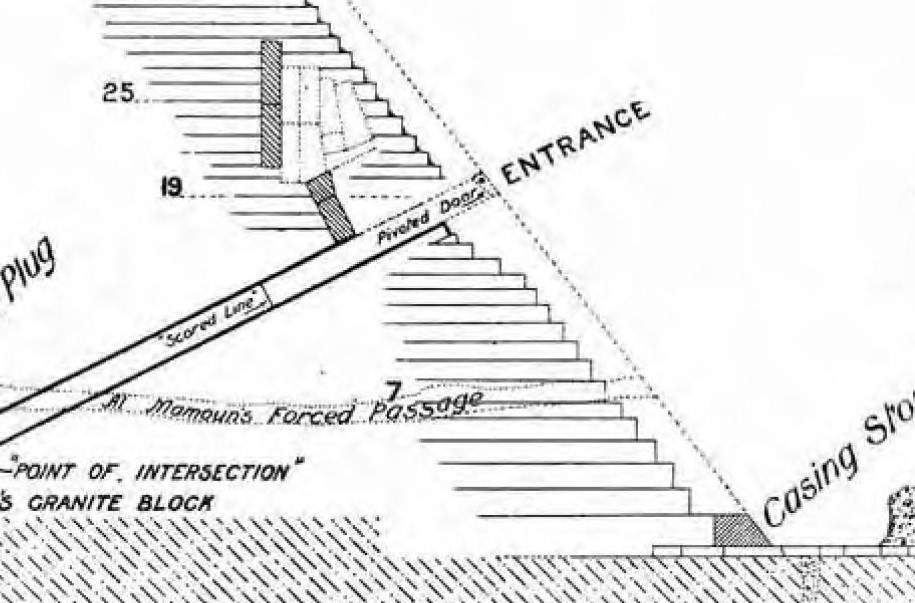
Hereunder is the well known drawing by Edgar. The chevron is on the right side just above the entrance.Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910; plate IX page 44.
Hereunder I have zoomed in.
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910; plate IX page 44. (Partly shown).
Two important details are revealed:
1. The lower part of the chevron is drawn just under the top of the 20th course.
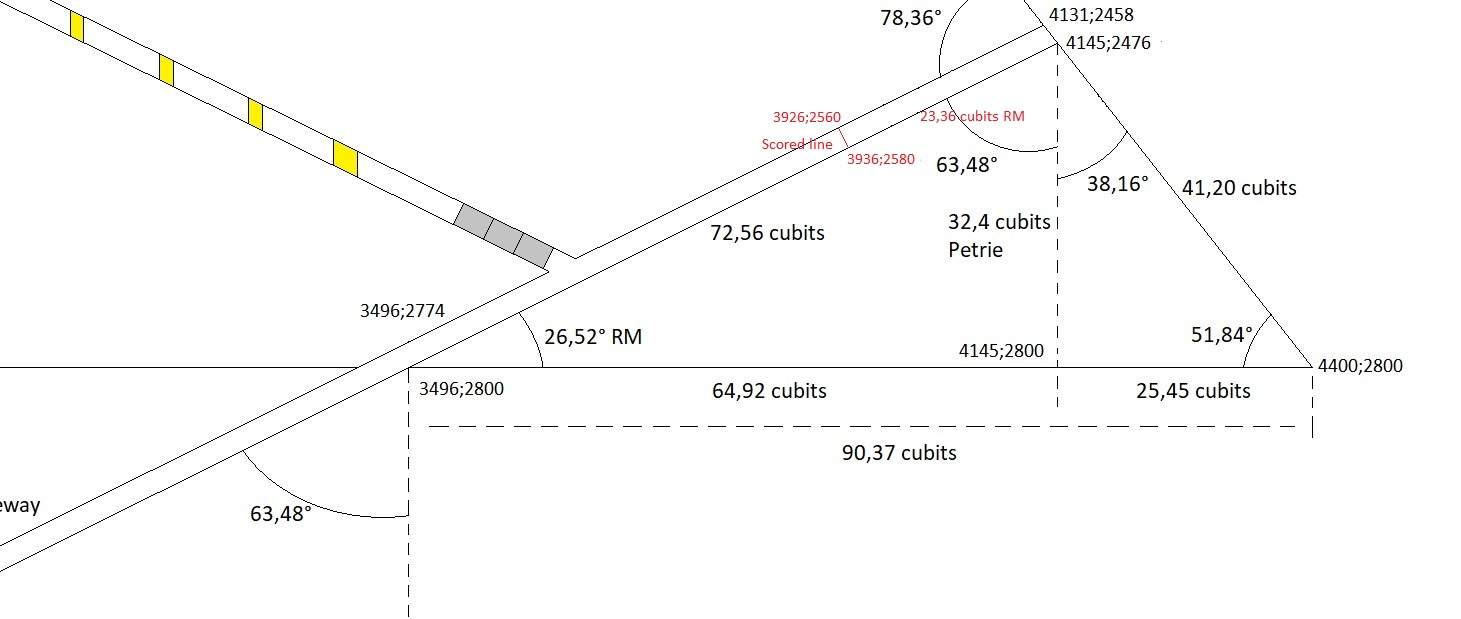
2. The "scored line" seems to hit the line of the roof of the descending passageway exactly under the southern part of the the midline of chevron, which means the cross points "F" or "G" added to the picture in section 5.1.3.
A coincidence ? Maybe not. The scored line might have been cut by the workers of the building as a marking point.
Section 5.1.5
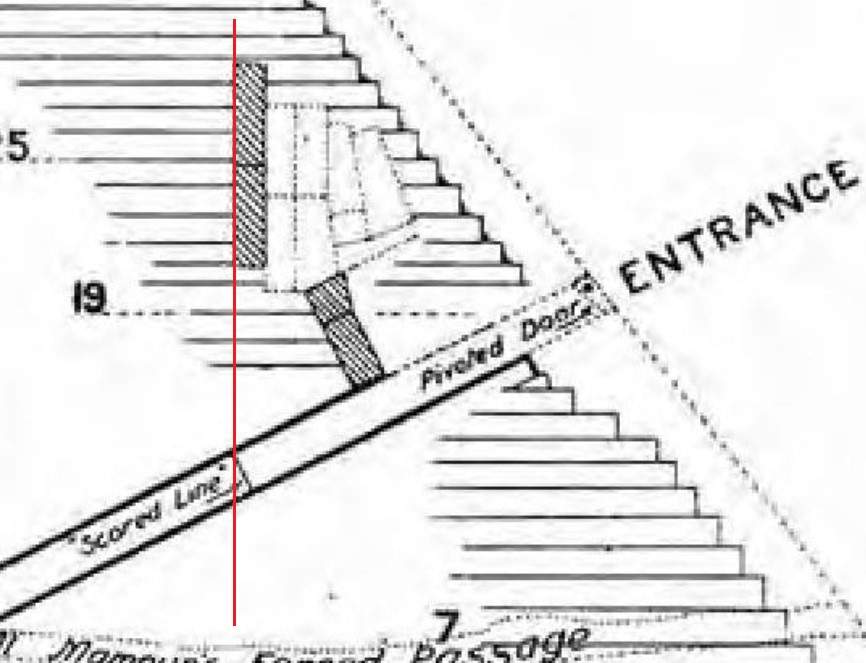
A vertical line is added to the drawing to verify the theory:
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910; plate IX page 44. (Partly shown and edited by me).
It seems the red line drawn from the back of the chevron hits the upper part of the scored line. Petrie measured the length from the original door to the scored line at floor level.
(S5orig-[S35]-P55-Line is marked in the table).
My comments:
481,59" = 23,36 cubits RM or 33,03 remens RM, (the ancient egyptians used sometimes remens instead of cubits for certain lengths).
From section 5.2.4 we know the height of the descending passageway perpendicular to the floor is 2,29 cubits RM and the floor of the original entrance begin at the coordinate 4145;2476.
The coordinate where the scored line hit the floor in descending passageway is:(4145 - 23,36 x 10 x cos 26,5231° ; 2476 + 23,36 x 10 x sin 26,5231° ) = 3936;2580
The scored line hit the roof at:
(3936 - 2,29 x 10 x sin 26,5231° ; 2580 - 2,29 x 10 x cos 26,5231° ) = 3926;2560
Section 5.1.6
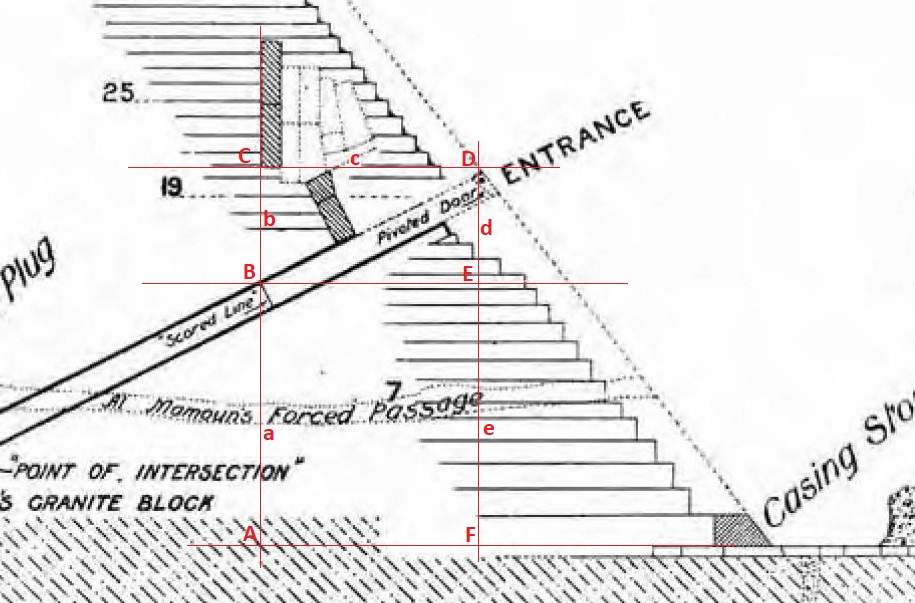
From the above mentioned red line drawn from the back of the chevron to the upper part of the scored line where it hits the roof of the descending passageway, we can calculate the height from the pavement to the lowest point of the midline of the chevron.
I have added more lines and capital letters at the cross points of the red lines. The small letters are distances between the cross points:Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910; plate IX page 44. (Partly shown and edited by me).
The reference length will therefore be the length from A to B, which is the length "a": (2800-2560) / 10 = 24,0 cubits.
The A and B cross points in the drawing have the coordinates:
A: 260;545 drw
B: 260;283 drw
The length "a" is 545 pixels - 283 pixels = 262 pixels
The factor between the pixels and cubits is: 24,0 cubits / 262 pixels = 0,0916 cubits/pixel.
Section 5.1.7
All the cross points have the following drawing coordinates:
A: 260;545 drw
B: 260;283 drw
C: 260;167 drw
D: 478;167 drw
E: 478;283 drw
F: 478;545 drw
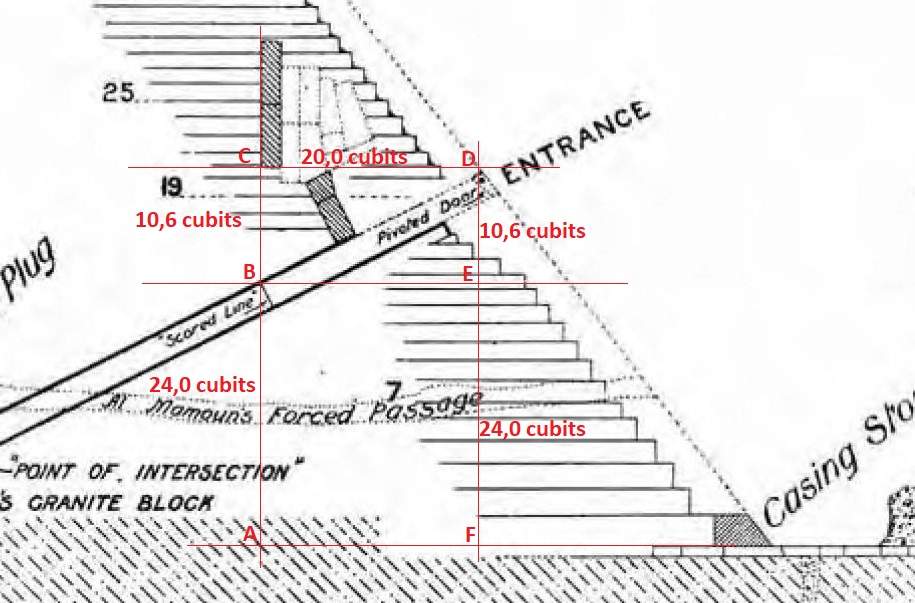
The lengths are:
"a": (545 pixels - 283 pixels) x 0,0916 cubits/pixels = 24,0 cubits.
"b": (283 pixels - 167 pixels) x 0,0916 cubits/pixels = 10,6 cubits.
"c": (478 pixels - 260 pixels) x 0,0916 cubits/pixels = 20,0 cubits.
"d": (283 pixels - 167 pixels) x 0,0916 cubits/pixels = 10,6 cubits.
"e": (545 pixels - 283 pixels) x 0,0916 cubits/pixels = 24,0 cubits
Section 5.1.8
The cubits are shown in the drawing:
Source: The Great Pyramid Passages And Chambers" Volume 1 by John and Morton Edgar, 1910; plate IX page 44. (Partly shown and edited by me).
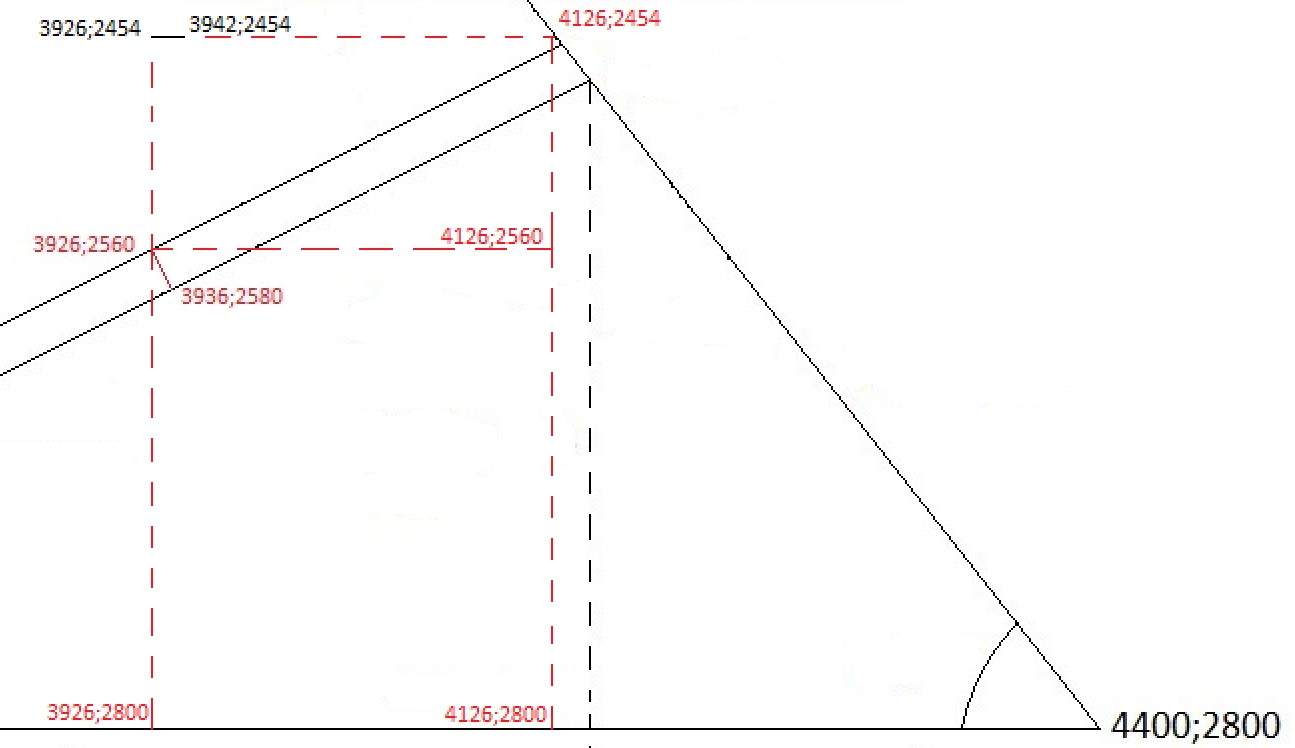
According to section 5.1.6, the master coordinate for cross point B is 3926;2560.
The cross point C is (3926;2560 - 10,6x10) = 3926;2454 , which is lowest southern point in the midline of the chevron.According to section 5.1.2, the thickness of the chevron is 33" or 1,60 cubits RM.
The lowest northern point in the midline of the chevron is (3926 + 1,6x10 ; 2454) = 3942;2454
Section 5.1.9
Master coordinates for cross points:
A: 260;545 drw : (3926; 2560 + 24,0x10) = 3926;2800 where the line hit the "Ground 0" level.
B: 260;283 drw :
3926;2560 Reference
C: 260;167 drw : (3926 ; 2560 - 10,6x10) = 3926;2454 which is lowest southern point in the midline of the chevron.
D: 478;167 drw : (3926 + 20,0x10 ; 2454 ) = 4126;2454 where the line hit the outer casing line.
E: 478;283 drw : (4126 ; 2454 + 10,6x10) = 4126;2560
F: 478;545 drw : (4126 ; 2560 + 24,0x10) = 4126;2800 where the line hit the "Ground 0" level.